Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yao, Jie
Chen, Xi
and
Hussain, Fazle
2019.
Reynolds number effect on drag control via spanwise wall oscillation in turbulent channel flows.
Physics of Fluids,
Vol. 31,
Issue. 8,
Yao, Jie
and
Hussain, Fazle
2020.
Turbulence statistics and coherent structures in compressible channel flow.
Physical Review Fluids,
Vol. 5,
Issue. 8,
Anbarlooei, H. R.
Cruz, D. O. A.
and
Ramos, F.
2020.
New power-law scaling for friction factor of extreme Reynolds number pipe flows.
Physics of Fluids,
Vol. 32,
Issue. 9,
Masud, Arif
and
Zhu, Lixing
2021.
Variationally derived closure models for large eddy simulation of incompressible turbulent flows.
International Journal for Numerical Methods in Fluids,
Vol. 93,
Issue. 7,
p.
2089.
Jiang, Chao
Vinuesa, Ricardo
Chen, Ruilin
Mi, Junyi
Laima, Shujin
and
Li, Hui
2021.
An interpretable framework of data-driven turbulence modeling using deep neural networks.
Physics of Fluids,
Vol. 33,
Issue. 5,
Xie, Jin-Han
de Silva, Charitha
Baidya, Rio
Yang, Xiang IA
and
Hu, Ruifeng
2021.
Third-order structure function in the logarithmic layer of boundary-layer turbulence.
Physical Review Fluids,
Vol. 6,
Issue. 7,
Motoori, Yutaro
and
Goto, Susumu
2021.
Hierarchy of coherent structures and real-space energy transfer in turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 911,
Issue. ,
Xie, Jiabin
He, Jianchao
Bao, Yun
and
Chen, Xi
2021.
A Low-Communication-Overhead Parallel DNS Method for the 3D Incompressible Wall Turbulence.
International Journal of Computational Fluid Dynamics,
Vol. 35,
Issue. 6,
p.
413.
Chen, Xi
and
Sreenivasan, Katepalli R.
2021.
Reynolds number scaling of the peak turbulence intensity in wall flows.
Journal of Fluid Mechanics,
Vol. 908,
Issue. ,
Birnir, Björn
Angheluta, Luiza
Kaminsky, John
and
Chen, Xi
2021.
Spectral link of the generalized Townsend-Perry constants in turbulent boundary layers.
Physical Review Research,
Vol. 3,
Issue. 4,
Wang, Limin
Hu, Ruifeng
and
Zheng, Xiaojing
2021.
A scaling improved inner–outer decomposition of near-wall turbulent motions.
Physics of Fluids,
Vol. 33,
Issue. 4,
Ruan, Joseph
and
Blanquart, Guillaume
2021.
Error estimation of a homogenized streamwise periodic boundary layer.
Physical Review Fluids,
Vol. 6,
Issue. 11,
Ji, Yong
Yao, Jie
Hussain, Fazle
and
Chen, Xi
2021.
Vorticity transports in turbulent channels under large-scale control via spanwise wall jet forcing.
Physics of Fluids,
Vol. 33,
Issue. 9,
Sanfins, G.
Anbarlooei, H. R.
Cruz, D. O. A.
and
Ramos, F.
2021.
Complete and incomplete similarity for the mean velocity profile of turbulent pipe and channel flows at extreme Reynolds number.
Physics of Fluids,
Vol. 33,
Issue. 8,
p.
085118.
Bi, Wei-Tao
Wei, Zhou
Zheng, Ke-Xin
and
She, Zhen-Su
2022.
A symmetry-based length model for characterizing the hypersonic boundary layer transition on a slender cone at moderate incidence.
Advances in Aerodynamics,
Vol. 4,
Issue. 1,
Chen, Yuandong
Wang, Xiaoning
Duan, Lishu
and
Wang, Jianchun
2022.
Effect of heat source on kinetic energy transfer in compressible homogeneous shear turbulence.
Physics of Fluids,
Vol. 34,
Issue. 12,
Yao, Jie
Chen, Xi
and
Hussain, Fazle
2022.
Direct numerical simulation of turbulent open channel flows at moderately high Reynolds numbers.
Journal of Fluid Mechanics,
Vol. 953,
Issue. ,
Chen, Xi
and
Sreenivasan, Katepalli R.
2022.
Law of bounded dissipation and its consequences in turbulent wall flows.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Ceci, Alessandro
Palumbo, Andrea
Larsson, Johan
and
Pirozzoli, Sergio
2022.
Numerical tripping of high-speed turbulent boundary layers.
Theoretical and Computational Fluid Dynamics,
Vol. 36,
Issue. 6,
p.
865.
Tang, Fan
and
Ji, Yong
2023.
A unified description of mean velocity in transitional- and turbulence-developed boundary layers.
AIP Advances,
Vol. 13,
Issue. 10,
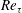 $Re_{\unicode[STIX]{x1D70F}}$) scaling transition of the maximum TMC located at
$Re_{\unicode[STIX]{x1D70F}}$) scaling transition of the maximum TMC located at 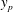 $y_{p}$. We show that in viscous units,
$y_{p}$. We show that in viscous units,  $y_{p}^{+}$ (and
$y_{p}^{+}$ (and 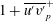 $1+\overline{u^{\prime }v^{\prime }}_{p}^{+}$) displays a scaling transition from
$1+\overline{u^{\prime }v^{\prime }}_{p}^{+}$) displays a scaling transition from 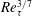 $Re_{\unicode[STIX]{x1D70F}}^{3/7}$ (
$Re_{\unicode[STIX]{x1D70F}}^{3/7}$ (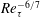 $Re_{\unicode[STIX]{x1D70F}}^{-6/7}$) to
$Re_{\unicode[STIX]{x1D70F}}^{-6/7}$) to  $Re_{\unicode[STIX]{x1D70F}}^{3/5}$ (
$Re_{\unicode[STIX]{x1D70F}}^{3/5}$ (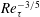 $Re_{\unicode[STIX]{x1D70F}}^{-3/5}$) in turbulent boundary layer, in sharp contrast to that from
$Re_{\unicode[STIX]{x1D70F}}^{-3/5}$) in turbulent boundary layer, in sharp contrast to that from  $Re_{\unicode[STIX]{x1D70F}}^{1/3}$ (
$Re_{\unicode[STIX]{x1D70F}}^{1/3}$ (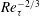 $Re_{\unicode[STIX]{x1D70F}}^{-2/3}$) to
$Re_{\unicode[STIX]{x1D70F}}^{-2/3}$) to  $Re_{\unicode[STIX]{x1D70F}}^{1/2}$ (
$Re_{\unicode[STIX]{x1D70F}}^{1/2}$ (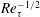 $Re_{\unicode[STIX]{x1D70F}}^{-1/2}$) in a channel/pipe, countering the prevailing view of a single universal near-wall scaling. This scaling transition reflects different near-wall motions in the buffer layer for small
$Re_{\unicode[STIX]{x1D70F}}^{-1/2}$) in a channel/pipe, countering the prevailing view of a single universal near-wall scaling. This scaling transition reflects different near-wall motions in the buffer layer for small 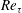 $Re_{\unicode[STIX]{x1D70F}}$ and log layer for large
$Re_{\unicode[STIX]{x1D70F}}$ and log layer for large 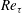 $Re_{\unicode[STIX]{x1D70F}}$, with the non-universality being ascribed to the presence/absence of mean wall-normal velocity
$Re_{\unicode[STIX]{x1D70F}}$, with the non-universality being ascribed to the presence/absence of mean wall-normal velocity 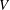 $V$. Our predictions are validated by a large set of data, and a probable flow state with a full coupling between momentum and energy cascades beyond a critical
$V$. Our predictions are validated by a large set of data, and a probable flow state with a full coupling between momentum and energy cascades beyond a critical  $Re_{\unicode[STIX]{x1D70F}}$ is envisaged.
$Re_{\unicode[STIX]{x1D70F}}$ is envisaged.
