Article contents
Model for thermal convection with uniform volumetric energy sources
Published online by Cambridge University Press: 25 May 2021
Abstract
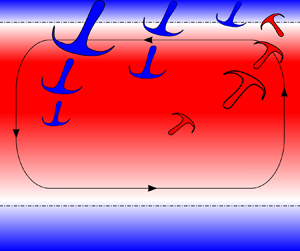
A theoretical model is derived to predict the heat fluxes at the upper and lower horizontal surfaces of an internally heated (IH) convection cell by extending the well-known Grossmann & Lohse (Phys. Rev. Lett., vol. 86, 2001, pp. 3316–3319) theory. The approach of Creyssels (J. Fluid Mech., vol. 900, 2020, p. A39) is generalized for a fluid heated internally and uniformly, confined between top and bottom plates of equal temperature. For each plate, a Nusselt number is defined and an analytical formula is given to predict its variations with the Rayleigh and Prandtl numbers. The turbulent flow produced in the upper half of the IH convection cell is very similar to that observed in standard Rayleigh–Bénard convection. On the contrary, the lower plate is swept by the large scale flow that circulates through the entire cell. The corresponding boundary layer is therefore modelled by a laminar boundary layer of the Blasius type. These predictions are consistent with the independent theoretical scalings proposed by Wang et al. (Geophys. Res. Lett., vol. 48, issue 4, 2021, p. e2020GL091198) and they are confirmed by the numerical results obtained by Goluskin & van der Poel (J. Fluid Mech., vol. 791, 2016, p. R6) and Wang et al. (Geophys. Res. Lett., vol. 48, issue 4, 2021, p. e2020GL091198).
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 4
- Cited by


