Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Facchini, Giulio
Favier, Benjamin
Le Gal, Patrice
Wang, Meng
and
Le Bars, Michael
2018.
The linear instability of the stratified plane Couette flow.
Journal of Fluid Mechanics,
Vol. 853,
Issue. ,
p.
205.
Xiao, Cheng-Nian
and
Senocak, Inanc
2019.
Stability of the Prandtl model for katabatic slope flows.
Journal of Fluid Mechanics,
Vol. 865,
Issue. ,
Senocak, Inanc
and
Xiao, Cheng-Nian
2020.
Modeling and Simulation of Turbulent Mixing and Reaction.
p.
47.
Dombrovsky, Leonid A.
Frenkel, Mark
Legchenkova, Irina
and
Bormashenko, Edward
2020.
Effect of thermal properties of a substrate on formation of self-arranged surface structures on evaporated polymer films.
International Journal of Heat and Mass Transfer,
Vol. 158,
Issue. ,
p.
120053.
Xiao, Cheng-Nian
and
Senocak, Inanc
2020.
Stability of the anabatic Prandtl slope flow in a stably stratified medium.
Journal of Fluid Mechanics,
Vol. 885,
Issue. ,
Robins, Luke J. M.
Kersalé, Evy
and
Jones, Chris A.
2020.
Viscous and inviscid strato-rotational instability.
Journal of Fluid Mechanics,
Vol. 894,
Issue. ,
Parente, E.
Robinet, J. C.
De Palma, P.
and
Cherubini, S.
2020.
Modal and nonmodal stability of a stably stratified boundary layer flow.
Physical Review Fluids,
Vol. 5,
Issue. 11,
Deguchi, Kengo
2021.
Eigenvalue bounds for compressible stratified magnetoshear flows varying in two transverse directions.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Le Gal, P.
Harlander, U.
Borcia, I. D.
Le Dizès, S.
Chen, J.
and
Favier, B.
2021.
Instability of vertically stratified horizontal plane Poiseuille flow.
Journal of Fluid Mechanics,
Vol. 907,
Issue. ,
Yalim, Jason
Welfert, Bruno D.
and
Lopez, Juan M.
2022.
Oblique instability of a stratified oscillatory boundary layer.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Xiao, Yue
Li, Yi
Zhao, Moli
and
Wang, Shaowei
2022.
Finite-amplitude instability of the buoyancy boundary layer in a thermally stratified medium.
Journal of Fluid Mechanics,
Vol. 947,
Issue. ,
Christin, Sarah
Meunier, Patrice
and
Le Dizès, Stéphane
2023.
Baroclinic critical layer in a viscous stratified boundary layer flow on an undulated tilted surface.
Journal of Fluid Mechanics,
Vol. 958,
Issue. ,
Lopez, Jose M.
Lopez, Juan M.
and
Marques, Francisco
2023.
Stably stratified Taylor–Couette flows.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2246,
Hamada, Gabriel Y.R.
Wolf, William R.
Pitz, Diogo B.
and
Alves, Leonardo S. de B.
2023.
Stability and receptivity analyses of mixed convection in unstably stratified horizontal boundary layers.
Journal of Fluid Mechanics,
Vol. 961,
Issue. ,
Lewin, Samuel F.
and
Caulfield, Colm-cille P.
2024.
Evidence for layered anisotropic stratified turbulence in a freely evolving horizontal shear flow.
Journal of Fluid Mechanics,
Vol. 983,
Issue. ,
Xiao, Cheng-Nian
and
Senocak, Inanc
2025.
Instabilities of speaker-wire vortices in katabatic Prandtl slope flows.
Physics of Fluids,
Vol. 37,
Issue. 1,
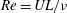 $Re=UL/{\it\nu}$ and the Froude number
$Re=UL/{\it\nu}$ and the Froude number 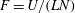 $F=U/(LN)$ where
$F=U/(LN)$ where 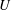 $U$ is the main stream velocity,
$U$ is the main stream velocity, 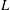 $L$ the boundary layer thickness,
$L$ the boundary layer thickness, 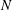 $N$ the buoyancy frequency and
$N$ the buoyancy frequency and  ${\it\nu}$ the kinematic viscosity. The diffusion of density is neglected. The boundary layer flow is found to be unstable with respect to two instabilities. The first one is the classical viscous instability which gives rise to Tollmien–Schlichting (TS) waves. We demonstrate that, even in the presence of stratification, the most unstable TS wave remains two-dimensional and therefore independent of the Froude number. The other instability is three-dimensional, inviscid in nature and associated with the stratification. It corresponds to the so-called radiative instability. We show that this instability appears first for
${\it\nu}$ the kinematic viscosity. The diffusion of density is neglected. The boundary layer flow is found to be unstable with respect to two instabilities. The first one is the classical viscous instability which gives rise to Tollmien–Schlichting (TS) waves. We demonstrate that, even in the presence of stratification, the most unstable TS wave remains two-dimensional and therefore independent of the Froude number. The other instability is three-dimensional, inviscid in nature and associated with the stratification. It corresponds to the so-called radiative instability. We show that this instability appears first for  $Re\geqslant Re_{c}^{(r)}\approx 1995$ for a Froude number close to 1.5 whereas the viscous instability develops for
$Re\geqslant Re_{c}^{(r)}\approx 1995$ for a Froude number close to 1.5 whereas the viscous instability develops for 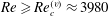 $Re\geqslant Re_{c}^{(v)}\approx 3980$. For large Reynolds numbers, the radiative instability is also shown to exhibit a much larger growth rate than the viscous instability in a large Froude number interval. We argue that this instability could develop in experimental facilities as well as in geophysical situations encountered in ocean and atmosphere.
$Re\geqslant Re_{c}^{(v)}\approx 3980$. For large Reynolds numbers, the radiative instability is also shown to exhibit a much larger growth rate than the viscous instability in a large Froude number interval. We argue that this instability could develop in experimental facilities as well as in geophysical situations encountered in ocean and atmosphere.