Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cao, Yong
and
Tamura, Tetsuro
2017.
Supercritical flows past a square cylinder with rounded corners.
Physics of Fluids,
Vol. 29,
Issue. 8,
Park, Doohyun
and
Yang, Kyung-Soo
2017.
Effect of rounding on flow-induced forces on a square cylinder.
Journal of Mechanical Science and Technology,
Vol. 31,
Issue. 12,
p.
5857.
Trip, Renzo
and
Fransson, Jens H. M.
2017.
Bluff body boundary-layer modification and its effect on the near-wake topology.
Physics of Fluids,
Vol. 29,
Issue. 9,
Yao, W.
and
Jaiman, R. K.
2017.
Model reduction and mechanism for the vortex-induced vibrations of bluff bodies.
Journal of Fluid Mechanics,
Vol. 827,
Issue. ,
p.
357.
Zheng, Qinmin
and
Alam, Md. Mahbub
2017.
Intrinsic features of flow past three square prisms in side-by-side arrangement.
Journal of Fluid Mechanics,
Vol. 826,
Issue. ,
p.
996.
Rastan, M. R.
Sohankar, A.
and
Alam, Md. Mahbub
2017.
Low-Reynolds-number flow around a wall-mounted square cylinder: Flow structures and onset of vortex shedding.
Physics of Fluids,
Vol. 29,
Issue. 10,
Bai, Honglei
and
Alam, Md. Mahbub
2018.
Dependence of square cylinder wake on Reynolds number.
Physics of Fluids,
Vol. 30,
Issue. 1,
Park, Doohyun
and
Yang, Kyung-Soo
2018.
Effects of planar shear on the three-dimensional instability in flow past a circular cylinder.
Physics of Fluids,
Vol. 30,
Issue. 3,
p.
034103.
Jiang, Hongyi
and
Cheng, Liang
2018.
Hydrodynamic characteristics of flow past a square cylinder at moderate Reynolds numbers.
Physics of Fluids,
Vol. 30,
Issue. 10,
Jiang, Hongyi
Cheng, Liang
and
An, Hongwei
2018.
Three-dimensional wake transition of a square cylinder.
Journal of Fluid Mechanics,
Vol. 842,
Issue. ,
p.
102.
Cao, Yong
and
Tamura, Tetsuro
2018.
Shear effects on flows past a square cylinder with rounded corners at Re=2.2×104.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 174,
Issue. ,
p.
119.
Sourav, Kumar
and
Sen, Subhankar
2019.
Transition of VIV-only motion of a square cylinder to combined VIV and galloping at low Reynolds numbers.
Ocean Engineering,
Vol. 187,
Issue. ,
p.
106208.
Arif, Md. Reyaz
and
Hasan, Nadeem
2019.
Vortex shedding suppression in mixed convective flow past a square cylinder subjected to large-scale heating using a non-Boussinesq model.
Physics of Fluids,
Vol. 31,
Issue. 2,
Zheng, Qinmin
and
Alam, Md. Mahbub
2019.
Evolution of the wake of three inline square prisms.
Physical Review Fluids,
Vol. 4,
Issue. 10,
Zafar, Farhan
and
Alam, Md. Mahbub
2019.
Flow structure around and heat transfer from cylinders modified from square to circular.
Physics of Fluids,
Vol. 31,
Issue. 8,
Wang, Rui
Zhu, Hongbo
Bao, Yan
Zhou, Dai
Ping, Huan
Han, Zhaolong
and
Xu, Hui
2019.
Modification of three-dimensional instability in the planar shear flow around two circular cylinders in tandem.
Physics of Fluids,
Vol. 31,
Issue. 10,
Li, Xintao
Lyu, Zhen
Kou, Jiaqing
and
Zhang, Weiwei
2019.
Mode competition in galloping of a square cylinder at low Reynolds number.
Journal of Fluid Mechanics,
Vol. 867,
Issue. ,
p.
516.
Miyanawala, T. P.
and
Jaiman, R. K.
2019.
Decomposition of wake dynamics in fluid–structure interaction via low-dimensional models.
Journal of Fluid Mechanics,
Vol. 867,
Issue. ,
p.
723.
Wang, Rui
Bao, Yan
Zhou, Dai
Zhu, Hongbo
Ping, Huan
Han, Zhaolong
Serson, Douglas
and
Xu, Hui
2019.
Flow instabilities in the wake of a circular cylinder with parallel dual splitter plates attached.
Journal of Fluid Mechanics,
Vol. 874,
Issue. ,
p.
299.
Zhang, Yuhang
Wang, Rui
Chen, Yaoran
Bao, Yan
Han, Zhaolong
Zhou, Dai
Ping, Huan
Fu, Shixiao
and
Zhao, Yongsheng
2020.
Three-dimensional wake transition in the flow over four square cylinders at low Reynolds numbers.
AIP Advances,
Vol. 10,
Issue. 1,
 $r$ in each edge of a square cylinder of height
$r$ in each edge of a square cylinder of height 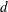 $d$ . Nine cases of rounding were considered, ranging from a square cylinder (
$d$ . Nine cases of rounding were considered, ranging from a square cylinder ( 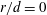 $r/d=0$ ) to a circular cylinder (
$r/d=0$ ) to a circular cylinder ( 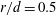 $r/d=0.5$ ) with an increment of 0.0625. Each cross-section was numerically implemented in a Cartesian grid system by using an immersed boundary method. The key parameters are the Reynolds number (Re) and the edge-radius ratio (
$r/d=0.5$ ) with an increment of 0.0625. Each cross-section was numerically implemented in a Cartesian grid system by using an immersed boundary method. The key parameters are the Reynolds number (Re) and the edge-radius ratio ( 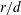 $r/d$ ). For low Re, the flow is steady and symmetric with respect to the centreline. Over the first critical Reynolds number (
$r/d$ ). For low Re, the flow is steady and symmetric with respect to the centreline. Over the first critical Reynolds number ( 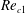 $Re_{c1}$ ), the flow undergoes a Hopf bifurcation to a time-periodic flow, termed the primary instability. As Re further increases, the onset of the secondary instability of three-dimensional (3D) nature is detected beyond the second critical Reynolds number (
$Re_{c1}$ ), the flow undergoes a Hopf bifurcation to a time-periodic flow, termed the primary instability. As Re further increases, the onset of the secondary instability of three-dimensional (3D) nature is detected beyond the second critical Reynolds number (  $Re_{c2}$ ). Rounding the sharp edges of a square cylinder significantly affects the flow topology, leading to noticeable changes in both instabilities. By employing the Stuart–Landau equation, we investigated the criticality of the primary instability depending upon
$Re_{c2}$ ). Rounding the sharp edges of a square cylinder significantly affects the flow topology, leading to noticeable changes in both instabilities. By employing the Stuart–Landau equation, we investigated the criticality of the primary instability depending upon  $r/d$ . The onset of the 3D secondary instability was detected by using Floquet stability analysis. The temporal and spatial characteristics of the dominant modes (A, B, QP) were described. The neutral stability curves of each mode were computed depending upon
$r/d$ . The onset of the 3D secondary instability was detected by using Floquet stability analysis. The temporal and spatial characteristics of the dominant modes (A, B, QP) were described. The neutral stability curves of each mode were computed depending upon 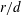 $r/d$ .
$r/d$ .

