Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sengupta, Tapan K.
Sengupta, Aditi
Sharma, Nidhi
Sengupta, Soumyo
Bhole, Ashish
and
Shruti, K. S.
2016.
Roles of bulk viscosity on Rayleigh-Taylor instability: Non-equilibrium thermodynamics due to spatio-temporal pressure fronts.
Physics of Fluids,
Vol. 28,
Issue. 9,
Sengupta, Tapan K
Sengupta, Aditi
Shruti, K S
Sengupta, Soumyo
and
Bhole, Ashish
2016.
Non-equilibrium Thermodynamics of Rayleigh-Taylor instability.
Journal of Physics: Conference Series,
Vol. 759,
Issue. ,
p.
012079.
Nagel, S. R.
Raman, K. S.
Huntington, C. M.
MacLaren, S. A.
Wang, P.
Barrios, M. A.
Baumann, T.
Bender, J. D.
Benedetti, L. R.
Doane, D. M.
Felker, S.
Fitzsimmons, P.
Flippo, K. A.
Holder, J. P.
Kaczala, D. N.
Perry, T. S.
Seugling, R. M.
Savage, L.
and
Zhou, Y.
2017.
A platform for studying the Rayleigh–Taylor and Richtmyer–Meshkov instabilities in a planar geometry at high energy density at the National Ignition Facility.
Physics of Plasmas,
Vol. 24,
Issue. 7,
Xie, C. Y.
Tao, J. J.
Sun, Z. L.
and
Li, J.
2017.
Retarding viscous Rayleigh-Taylor mixing by an optimized additional mode.
Physical Review E,
Vol. 95,
Issue. 2,
Zhou, Ye
2017.
Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. I.
Physics Reports,
Vol. 720-722,
Issue. ,
p.
1.
Elbaz, Yonatan
and
Shvarts, Dov
2018.
Modal model mean field self-similar solutions to the asymptotic evolution of Rayleigh-Taylor and Richtmyer-Meshkov instabilities and its dependence on the initial conditions.
Physics of Plasmas,
Vol. 25,
Issue. 6,
Shimony, Assaf
Malamud, Guy
and
Shvarts, Dov
2018.
Density Ratio and Entrainment Effects on Asymptotic Rayleigh–Taylor Instability.
Journal of Fluids Engineering,
Vol. 140,
Issue. 5,
Zeng, Qingyun
Gonzalez-Avila, Silvestre Roberto
Voorde, Sophie Ten
and
Ohl, Claus-Dieter
2018.
Jetting of viscous droplets from cavitation-induced Rayleigh–Taylor instability.
Journal of Fluid Mechanics,
Vol. 846,
Issue. ,
p.
916.
Belova, A. O.
Lymar, V. I.
Makovetskyi, Ye. D.
Pogrebnyak, M. L.
and
Rudenko, A. S.
2019.
Threshold Behavior of Convective Flows at Thermal Lens Oscillations.
p.
498.
Kord, Ali
and
Capecelatro, Jesse
2019.
Optimal perturbations for controlling the growth of a Rayleigh–Taylor instability.
Journal of Fluid Mechanics,
Vol. 876,
Issue. ,
p.
150.
Zhou, Ye
Clark, Timothy T.
Clark, Daniel S.
Gail Glendinning, S.
Aaron Skinner, M.
Huntington, Channing M.
Hurricane, Omar A.
Dimits, Andris M.
and
Remington, Bruce A.
2019.
Turbulent mixing and transition criteria of flows induced by hydrodynamic instabilities.
Physics of Plasmas,
Vol. 26,
Issue. 8,
Chen, Jun
Shen, Chao-Qun
Wang, He
and
Zhang, Cheng-Bin
2019.
Rayleigh-Bénard-Marangoni convection characteristics during mass transfer between liquid layers.
Acta Physica Sinica,
Vol. 68,
Issue. 7,
p.
074701.
Zhou, Ye
and
Cabot, William H.
2019.
Time-dependent study of anisotropy in Rayleigh-Taylor instability induced turbulent flows with a variety of density ratios.
Physics of Fluids,
Vol. 31,
Issue. 8,
de Freitas Duarte, Bernardo Alan
Villar, Millena Martins
Serfaty, Ricardo
and
da Silveira Neto, Aristeu
2019.
Evaluation of a diffuse interface treatment for pressure in phase change simulations using adaptive mesh refinement.
Journal of the Brazilian Society of Mechanical Sciences and Engineering,
Vol. 41,
Issue. 2,
Zhang, You-sheng
Ruan, Yu-cang
Xie, Han-song
and
Tian, Bao-lin
2020.
Mixed mass of classical Rayleigh-Taylor mixing at arbitrary density ratios.
Physics of Fluids,
Vol. 32,
Issue. 1,
Livescu, Daniel
2020.
Turbulence with Large Thermal and Compositional Density Variations.
Annual Review of Fluid Mechanics,
Vol. 52,
Issue. 1,
p.
309.
Zhang, You-sheng
He, Zhi-wei
Xie, Han-song
Xiao, Meng-Juan
and
Tian, Bao-lin
2020.
Methodology for determining coefficients of turbulent mixing model.
Journal of Fluid Mechanics,
Vol. 905,
Issue. ,
Do, Alexandre
Pickworth, Louisa A.
Kozioziemski, Bernard J.
Angulo, Adrianna M.
Hall, Gareth N.
Nagel, Sabrina R.
Bradley, David K.
Mccarville, Tom
and
Ayers, Jay M.
2020.
Fresnel zone plate development for x-ray radiography of hydrodynamic instabilities at the National Ignition Facility.
Applied Optics,
Vol. 59,
Issue. 34,
p.
10777.
Ruan, Yu-cang
Zhang, You-sheng
Tian, Bao-lin
and
Zhang, Xin-ting
2020.
Density-ratio-invariant mean-species profile of classical Rayleigh-Taylor mixing.
Physical Review Fluids,
Vol. 5,
Issue. 5,
Fan, Zhengfeng
and
Dong, Ming
2020.
Multiple eigenmodes of the Rayleigh-Taylor instability observed for a fluid interface with smoothly varying density. III. Excitation and nonlinear evolution.
Physical Review E,
Vol. 101,
Issue. 6,
 $A\equiv ({\it\rho}_{2}-{\it\rho}_{1})/({\it\rho}_{2}+{\it\rho}_{1})=0.48$ . The liquid-filled tank is attached to a test sled that is accelerated downwards along a vertical rail system using a system of weights and pulleys producing approximately
$A\equiv ({\it\rho}_{2}-{\it\rho}_{1})/({\it\rho}_{2}+{\it\rho}_{1})=0.48$ . The liquid-filled tank is attached to a test sled that is accelerated downwards along a vertical rail system using a system of weights and pulleys producing approximately  $1g$ net acceleration. The tank is backlit and images are digitally recorded using a high-speed video camera. The experiments are either initiated with forced initial perturbations or are left unforced. The forced experiments have an initial perturbation imposed by vertically oscillating the liquid-filled tank to produce Faraday waves at the interface. The unforced experiments rely on random interfacial fluctuations, resulting from background noise, to seed the instability. The main focus of this study is to determine the effects of forced initial perturbations and the effects of miscibility on the growth parameter,
$1g$ net acceleration. The tank is backlit and images are digitally recorded using a high-speed video camera. The experiments are either initiated with forced initial perturbations or are left unforced. The forced experiments have an initial perturbation imposed by vertically oscillating the liquid-filled tank to produce Faraday waves at the interface. The unforced experiments rely on random interfacial fluctuations, resulting from background noise, to seed the instability. The main focus of this study is to determine the effects of forced initial perturbations and the effects of miscibility on the growth parameter,  ${\it\alpha}$ . Measurements of the mixing-layer width,
${\it\alpha}$ . Measurements of the mixing-layer width, 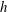 $h$ , are acquired, from which
$h$ , are acquired, from which  ${\it\alpha}$ is determined. It is found that initial perturbations of the form used in this study do not affect measured
${\it\alpha}$ is determined. It is found that initial perturbations of the form used in this study do not affect measured  ${\it\alpha}$ values. However, miscibility is observed to strongly affect
${\it\alpha}$ values. However, miscibility is observed to strongly affect  ${\it\alpha}$ , resulting in a factor of two reduction in its value, a finding not previously observed in past experiments. In addition, all measured
${\it\alpha}$ , resulting in a factor of two reduction in its value, a finding not previously observed in past experiments. In addition, all measured  ${\it\alpha}$ values are found to be smaller than those obtained in previous experimental studies.
${\it\alpha}$ values are found to be smaller than those obtained in previous experimental studies.

