Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Song, Jiaxing
Lin, Fenghui
Zhu, Yabiao
Wan, Zhen-Hua
Liu, Nansheng
Lu, Xi-Yun
and
Khomami, Bamin
2023.
Self-sustaining cycle of purely elastic turbulence.
Physical Review Fluids,
Vol. 8,
Issue. 1,
Bai, Yang
Latrache, Noureddine
Kelai, Fayçal
Crumeyrolle, Olivier
and
Mutabazi, Innocent
2023.
Viscoelastic instabilities of Taylor–Couette flows with different rotation regimes.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2246,
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2023.
Experimental insights into elasto-inertial transitions in Taylor–Couette flows.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2243,
2023.
Effects of polymer additives on the entrainment of turbulent water jet.
Physics of Fluids,
Vol. 35,
Issue. 4,
Song, Jiaxing
Zhu, Yabiao
Lin, Fenghui
Liu, Nansheng
and
Khomami, Bamin
2023.
Turbulent Taylor–Couette flow of dilute polymeric solutions: a 10-year retrospective.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2243,
Foggi Rota, Giulio
Amor, Christian
Le Clainche, Soledad
and
Rosti, Marco Edoardo
2024.
Unified view of elastic and elasto-inertial turbulence in channel flows at low and moderate Reynolds numbers.
Physical Review Fluids,
Vol. 9,
Issue. 12,
Zhang, Jing-yang
Wang, Ren-xuan
Lyu, Yuan-wei
Huang, Pu
Zhang, Jing-zhou
Huang, Zhong-wen
and
Cheng, Feng-na
2024.
Thermal effect induced by viscous dissipation on characteristics of aerodynamic foil journal bearing with aero-thermo-elastic coupling.
Thermal Science and Engineering Progress,
Vol. 50,
Issue. ,
p.
102511.
Otto, Samuel E.
Oishi, Cassio M.
Amaral, Fabio V.G.
Brunton, Steven L.
and
Nathan Kutz, J.
2024.
Machine learning in viscoelastic fluids via energy-based kernel embedding.
Journal of Computational Physics,
Vol. 516,
Issue. ,
p.
113371.
Lin, Fenghui
Song, Jiaxing
Liu, Nansheng
Wan, Zhenhua
Lu, Xi-Yun
and
Khomami, Bamin
2024.
Maximum drag enhancement asymptote in turbulent Taylor–Couette flow of dilute polymeric solutions.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 323,
Issue. ,
p.
105172.
Garg, Himani
and
Wang, Lei
2024.
Enhanced heat transfer in a two-dimensional serpentine micro-channel using elastic polymers.
International Journal of Thermofluids,
Vol. 23,
Issue. ,
p.
100724.
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2024.
Coherent structures of elastoinertial instabilities in Taylor–Couette flows.
Journal of Fluid Mechanics,
Vol. 986,
Issue. ,
Yerasi, Sumithra R.
Picardo, Jason R.
Gupta, Anupam
and
Vincenzi, Dario
2024.
Preserving large-scale features in simulations of elastic turbulence.
Journal of Fluid Mechanics,
Vol. 1000,
Issue. ,
Xu, Chang
Zhang, Chengyao
Brandt, Luca
Song, Jiaxing
and
Shishkina, Olga
2025.
Direct numerical simulations of turbulent Rayleigh–Bénard convection with polymer additives.
Journal of Fluid Mechanics,
Vol. 1014,
Issue. ,
Surya Phani Tej, P. S. D.
Kumar Mohanty, Pratyush
and
Shankar, V.
2025.
Hoop stress and polymer diffusive instabilities in viscoelastic Taylor–Couette flow.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 481,
Issue. 2321,
Lin, Fenghui
Liao, Zi-Mo
Zhao, Zhiye
Liu, Nansheng
Lu, Xi-Yun
and
Khomami, Bamin
2025.
GPU acceleration of a hi-fidelity algorithm for direct numerical simulation of polymer-induced/modified turbulence.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 342,
Issue. ,
p.
105437.
Adibi, Tohid
Razavi, Seyed Esmail
Ahmed, Shams Forruque
Bonab, Parvaneh Alizadeh
Debnath, J. C.
and
Alotaibi, Hammad
2025.
Numerical Investigation of Turbulent Mixed Convection Between Coaxial Cylinders and the Inner Rotating Cylinder With Axial Flow.
Energy Science & Engineering,
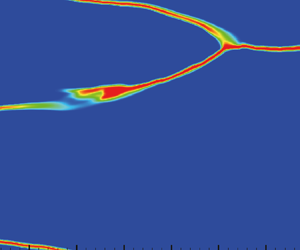
 $Re$) over seven orders of magnitude, i.e. from
$Re$) over seven orders of magnitude, i.e. from  $Re=1000$ to
$Re=1000$ to  $0.0001$. The dominant spatio-temporal flow features in the elastic turbulence regime are those of large-scale unsteady diwhirls and small-scale axial and azimuthal travelling waves in the outer and inner halves of the gap, respectively. Moreover, it is conclusively shown that production of turbulent kinetic energy in purely elastic turbulence solely arises due to the stochastic nature of polymer stretch/relaxation. Overall, based on this comprehensive numerical investigation, the differences in the underlying fluid physics that give rise to turbulent fluctuations in elastically dominated and purely elastic turbulence have been delineated.
$0.0001$. The dominant spatio-temporal flow features in the elastic turbulence regime are those of large-scale unsteady diwhirls and small-scale axial and azimuthal travelling waves in the outer and inner halves of the gap, respectively. Moreover, it is conclusively shown that production of turbulent kinetic energy in purely elastic turbulence solely arises due to the stochastic nature of polymer stretch/relaxation. Overall, based on this comprehensive numerical investigation, the differences in the underlying fluid physics that give rise to turbulent fluctuations in elastically dominated and purely elastic turbulence have been delineated.

