Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yang, Xiao
Li, Xiao-Ming
Kong, Qing-Qiang
Liu, Zhuo
Chen, Jing-Peng
Jia, Hui
Liu, Yan-Zhen
Xie, Li-Jing
and
Chen, Cheng-Meng
2020.
One-pot ball-milling preparation of graphene/carbon black aqueous inks for highly conductive and flexible printed electronics.
Science China Materials,
Vol. 63,
Issue. 3,
p.
392.
Wu, Zhihu
Hao, Jiguang
Lu, Jie
Xu, Long
Hu, Gengkai
and
Floryan, J. M.
2020.
Small droplet bouncing on a deep pool.
Physics of Fluids,
Vol. 32,
Issue. 1,
Antonopoulou, E.
Harlen, O. G.
Walkley, M. A.
and
Kapur, N.
2020.
Jetting behavior in drop-on-demand printing: Laboratory experiments and numerical simulations.
Physical Review Fluids,
Vol. 5,
Issue. 4,
Chang, Jiaqing
Jiang, Fan
Liu, Ziteng
Zhu, Dachang
and
Shen, Teng
2021.
Simulation and experimental study on droplet breakup modes and redrawing of their phase diagram.
Physics of Fluids,
Vol. 33,
Issue. 8,
Hu, Liang
She, Lei
Fang, Yanshen
Su, Rui
and
Fu, Xin
2021.
Deformation characteristics of droplet generated by Rayleigh jet breakup.
AIP Advances,
Vol. 11,
Issue. 4,
Almusallam, Abdulwahab S.
and
Bini, T. B.
2021.
Modeling vorticity stretching of viscoelastic droplets during shearing flow.
Journal of Rheology,
Vol. 65,
Issue. 6,
p.
1327.
Shah, Muhammad Ali
Lee, Duck-Gyu
Lee, Bo-Yeon
and
Hur, Shin
2021.
Classifications and Applications of Inkjet Printing Technology: A Review.
IEEE Access,
Vol. 9,
Issue. ,
p.
140079.
Jiao, Tian
Lian, Qin
Zhao, Tingze
and
Wang, Huichao
2021.
Influence of ink properties and voltage parameters on piezoelectric inkjet droplet formation.
Applied Physics A,
Vol. 127,
Issue. 1,
Wang, Liping
Kong, Weiliang
Bian, Peixiang
Wang, Fuxin
and
Liu, Hong
2022.
Experimental investigation on the performances of a valve-based and on-demand droplet generator producing droplets in a wide size range.
AIP Advances,
Vol. 12,
Issue. 9,
Wang, Li
Fu, Haojie
Zhang, Zhaofa
Wang, Jiajian
Feng, Jiahua
and
Lu, Bingheng
2022.
Development of high-frequency and high-viscosity piezoelectric DOD print head and its jet performance.
Sensors and Actuators A: Physical,
Vol. 337,
Issue. ,
p.
113409.
Li, Dege
Li, Haoren
Yang, Guodong
Wang, Jide
Huang, Bingfang
Wu, Xinlei
Sun, Qiang
Ma, Chi
Liu, Yonghong
and
Zhang, Yanzhen
2022.
Subharmonic resonance and antiresonance characteristics for high-frequency confined interface vibration inkjet printing.
Physics of Fluids,
Vol. 34,
Issue. 3,
Khobaib, Khobaib
Rozynek, Zbigniew
and
Hornowski, Tomasz
2022.
Mechanical properties of particle-covered droplets probed by nonuniform electric field.
Journal of Molecular Liquids,
Vol. 354,
Issue. ,
p.
118834.
Yang, Zhengjie
Tian, Hongmiao
Wang, Chunhui
Li, Xiangming
Chen, Xiaoliang
Chen, Xiaoming
and
Shao, Jinyou
2022.
Actuation waveform optimization via multi-pulse crosstalk modulation for stable ultra-high frequency piezoelectric drop-on-demand printing.
Additive Manufacturing,
Vol. 60,
Issue. ,
p.
103165.
Lohse, Detlef
2022.
Fundamental Fluid Dynamics Challenges in Inkjet Printing.
Annual Review of Fluid Mechanics,
Vol. 54,
Issue. 1,
p.
349.
Li, Dege
Li, Haoren
Yang, Guodong
Cao, Yi
Huang, Bingfang
Wu, Xinlei
Sun, Qiang
Ma, Chi
Zhou, Yu
Liu, Yonghong
and
Zhang, Yanzhen
2022.
Mechanisms of inkjet printing in a liquid environment.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Li, Gang
Liu, Tingting
Xiao, Xingzhi
Gu, Mingfei
and
Liao, Wenhe
2022.
Numerical simulations of droplet forming, breaking and depositing behaviors in high-viscosity paste jetting.
Journal of Manufacturing Processes,
Vol. 78,
Issue. ,
p.
172.
Yesuf, Hanur Meku
Jhatial, Abdul Khalique
Gianchandani, Pardeep Kumar
Siddique, Amna
and
Simair, Altaf Ahmed
2023.
Digital Textile Printing.
p.
21.
Tang, Shengchang
Wei, Xiangfu
Huang, Guangyong
Dai, Peng
Cai, Yongchao
Sun, Cuimin
and
You, Hui
2024.
Analysis of drop-on-demand printing characteristics and stability driven by inertial forces.
Physics of Fluids,
Vol. 36,
Issue. 7,
Lin, Xiao-Chen
Wang, Hai-Xing
Tian, Sheng-Wei
Geng, Jin-Yue
Huang, He-Ji
Sun, Su-Rong
Cao, Jin-Wen
Meng, Xian
and
Yan, Cong
2024.
Numerical study on the effect of actuation parameters on the formation characteristics of metal droplets in magnetohydrodynamic drop-on-demand (DOD) jetting.
Journal of Materials Processing Technology,
Vol. 330,
Issue. ,
p.
118483.
Li, Zihao
Zhang, Yanzhen
Li, Dege
Wang, Jide
Xiang, Teng
He, Weiwei
Hu, Guofang
Wu, Yuyao
Xue, Boce
and
Li, Runsheng
2024.
High-frequency electrohydrodynamic jet printing based on meniscus vibration.
Physics of Fluids,
Vol. 36,
Issue. 12,
 $T$ and a decreasing amplitude as the droplet moves downwards. The deformation extent is found strongly dependent on the capillary number (
$T$ and a decreasing amplitude as the droplet moves downwards. The deformation extent is found strongly dependent on the capillary number (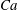 $Ca$), first ascending and then descending as the number increases. The maximum value of the deformation extent is surprisingly found to be within range of 0.068–0.082 of the
$Ca$), first ascending and then descending as the number increases. The maximum value of the deformation extent is surprisingly found to be within range of 0.068–0.082 of the 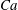 $Ca$ number regardless of other factors. Furthermore, the Rayleigh’s linear relation of the oscillation frequency of the droplet to the parameter,
$Ca$ number regardless of other factors. Furthermore, the Rayleigh’s linear relation of the oscillation frequency of the droplet to the parameter, 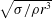 $\sqrt{\unicode[STIX]{x1D70E}/\unicode[STIX]{x1D70C}r^{3}}$ (where
$\sqrt{\unicode[STIX]{x1D70E}/\unicode[STIX]{x1D70C}r^{3}}$ (where  $\unicode[STIX]{x1D70E}$ is the surface tension coefficient,
$\unicode[STIX]{x1D70E}$ is the surface tension coefficient, 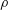 $\unicode[STIX]{x1D70C}$ is the density and
$\unicode[STIX]{x1D70C}$ is the density and  $r$ is the droplet’s radius), is updated with a smaller slope shown both by experiment and simulation.
$r$ is the droplet’s radius), is updated with a smaller slope shown both by experiment and simulation.
