Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Voronka, Roman
and
Keller, Joseph B.
1975.
Asymptotic analysis of stochastic models in population genetics.
Mathematical Biosciences,
Vol. 25,
Issue. 3-4,
p.
331.
Smith, Truett L.
and
Tsokos, Chris P.
1976.
On approximating the expected behavior of stochastic epidemiological models applicable to small populations.
Applied Mathematics and Computation,
Vol. 2,
Issue. 2,
p.
95.
Bailey, Norman T.J.
1986.
Macro-modelling and prediction of epidemic spread at community level.
Mathematical Modelling,
Vol. 7,
Issue. 5-8,
p.
689.
Isham, Valerie
1991.
Assessing the variability of stochastic epidemics.
Mathematical Biosciences,
Vol. 107,
Issue. 2,
p.
209.
Azaele, Sandro
Maritan, Amos
Bertuzzo, Enrico
Rodriguez-Iturbe, Ignacio
and
Rinaldo, Andrea
2010.
Stochastic dynamics of cholera epidemics.
Physical Review E,
Vol. 81,
Issue. 5,

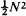 equations to be solved for the general stochastic epidemic while the number of equations in the approximating system is proportional to
equations to be solved for the general stochastic epidemic while the number of equations in the approximating system is proportional to 