Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ghurye, S. G.
1987.
Some multivariate lifetime distributions.
Advances in Applied Probability,
Vol. 19,
Issue. 01,
p.
138.
Sarkar, Sanat K.
1987.
A Continuous Bivariate Exponential Distribution.
Journal of the American Statistical Association,
Vol. 82,
Issue. 398,
p.
667.
Ghurye, S. G.
1987.
Some multivariate lifetime distributions.
Advances in Applied Probability,
Vol. 19,
Issue. 1,
p.
138.
Marshall, Albert W.
and
Olkin, Ingram
1991.
Functional equations for multivariate exponential distributions.
Journal of Multivariate Analysis,
Vol. 39,
Issue. 1,
p.
209.
1997.
Multivariate Models and Multivariate Dependence Concepts.
Vol. 19970691,
Issue. ,
LI, ZEHUI
CHAN, LING-YAU
and
YUAN, ZHIXIN
1999.
FAILURE TIME DISTRIBUTION UNDER A δ-SHOCK MODEL AND ITS APPLICATION TO ECONOMIC DESIGN OF SYSTEMS.
International Journal of Reliability, Quality and Safety Engineering,
Vol. 06,
Issue. 03,
p.
237.
Fan, Juanjuan
Ghurye, S. G.
and
Levine, Richard A.
2000.
Multicomponent lifetime distributions in the presence of ageing.
Journal of Applied Probability,
Vol. 37,
Issue. 2,
p.
521.
Kulkarni, H. V.
2006.
Characterizations and Modelling of Multivariate Lack of Memory Property.
Metrika,
Vol. 64,
Issue. 2,
p.
167.
Kulkarni, H. V.
and
Patkure, B. B.
2007.
A New Variant of the Bivariate Setting the Clock Back to Zero Property.
Communications in Statistics - Theory and Methods,
Vol. 36,
Issue. 13,
p.
2339.
Hanagal, David D.
and
Mandrekar, V.
2009.
Generalized Loss of Memory Property and a Multivariate Extension.
Economic Quality Control,
Vol. 24,
Issue. 1,
Balakrishna, N.
and
Lai, Chin Diew
2009.
Continuous Bivariate Distributions.
p.
401.
Mulero, Julio
and
Pellerey, Franco
2010.
Bivariate Aging Properties under Archimedean Dependence Structures.
Communications in Statistics - Theory and Methods,
Vol. 39,
Issue. 17,
p.
3108.
Li, Xiaohu
and
Pellerey, Franco
2011.
Generalized Marshall–Olkin distributions and related bivariate aging properties.
Journal of Multivariate Analysis,
Vol. 102,
Issue. 10,
p.
1399.
Lin, Gwo Dong
Dou, Xiaoling
and
Kuriki, Satoshi
2019.
The Bivariate Lack-of-Memory Distributions.
Sankhya A,
Vol. 81,
Issue. 2,
p.
273.
Gobbi, Fabio
Kolev, Nikolai
and
Mulinacci, Sabrina
2021.
Ryu-type extended Marshall-Olkin model with implicit shocks and joint life insurance applications.
Insurance: Mathematics and Economics,
Vol. 101,
Issue. ,
p.
342.
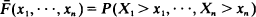 satisfies the functional equation
satisfies the functional equation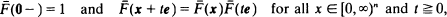
 is the multivariate exponential distribution of Marshall and Olkin. The functional equation has many solutions if the requirement of exponential marginals is not imposed, but the class of possible marginals is somewhat limited (e.g., marginals must be absolutely continuous). The class of possible solutions of the equation is characterized in this paper, and several examples are obtained from models for dependence that may be of practical interest.
is the multivariate exponential distribution of Marshall and Olkin. The functional equation has many solutions if the requirement of exponential marginals is not imposed, but the class of possible marginals is somewhat limited (e.g., marginals must be absolutely continuous). The class of possible solutions of the equation is characterized in this paper, and several examples are obtained from models for dependence that may be of practical interest.
