Published online by Cambridge University Press: 17 May 2022
The Chow–Robbins game is a classical, still partly unsolved, stopping problem introduced by Chow and Robbins in 1965. You repeatedly toss a fair coin. After each toss, you decide whether you take the fraction of heads up to now as a payoff, otherwise you continue. As a more general stopping problem this reads  $V(n,x) = \sup_{\tau }\mathbb{E} \left [ \frac{x + S_\tau}{n+\tau}\right]$
, where S is a random walk. We give a tight upper bound for V when S has sub-Gaussian increments by using the analogous time-continuous problem with a standard Brownian motion as the driving process. For the Chow–Robbins game we also give a tight lower bound and use these to calculate, on the integers, the complete continuation and the stopping set of the problem for
$V(n,x) = \sup_{\tau }\mathbb{E} \left [ \frac{x + S_\tau}{n+\tau}\right]$
, where S is a random walk. We give a tight upper bound for V when S has sub-Gaussian increments by using the analogous time-continuous problem with a standard Brownian motion as the driving process. For the Chow–Robbins game we also give a tight lower bound and use these to calculate, on the integers, the complete continuation and the stopping set of the problem for 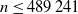 $n\leq 489\,241$
.
$n\leq 489\,241$
.