Article contents
On passage and conditional passage times for Markov chains in continuous time
Published online by Cambridge University Press: 14 July 2016
Abstract
Let X(t) be a temporally homogeneous irreducible Markov chain in continuous time defined on 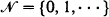 . For k < i < j, let H = {k + 1, ···, j − 1} and let kTij (jTik ) be the upward (downward) conditional first-passage time of X(t) from i to j(k) given no visit to
. For k < i < j, let H = {k + 1, ···, j − 1} and let kTij (jTik ) be the upward (downward) conditional first-passage time of X(t) from i to j(k) given no visit to 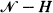 . These conditional passage times are studied through first-passage times of a modified chain HX(t) constructed by making the set
. These conditional passage times are studied through first-passage times of a modified chain HX(t) constructed by making the set  of states absorbing. It will be shown that the densities of kTij and jTik for any birth-death process are unimodal and the modes kmij (jmik ) of the unimodal densities are non-increasing (non-decreasing) with respect to i. Some distribution properties of kTij and jTik for a time-reversible Markov chain are presented. Symmetry among kTij, jTik ,
of states absorbing. It will be shown that the densities of kTij and jTik for any birth-death process are unimodal and the modes kmij (jmik ) of the unimodal densities are non-increasing (non-decreasing) with respect to i. Some distribution properties of kTij and jTik for a time-reversible Markov chain are presented. Symmetry among kTij, jTik , 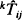 and
and  is also discussed, where
is also discussed, where 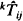 , and
, and  are conditional passage times of the reversed process of X(t).
are conditional passage times of the reversed process of X(t).
Information
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1988
References
- 3
- Cited by

