Published online by Cambridge University Press: 14 July 2016
Consider a compact body E embedded in a convex compact body G. A point P is randomly chosen in E and three different rays to the boundary ∂G of G are generated by P. Ray 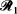 is in a uniformly random direction and has length R, ray
is in a uniformly random direction and has length R, ray 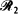 is through a second random point chosen from within G and has length W, and ray
is through a second random point chosen from within G and has length W, and ray 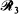 is to a random point in ∂G and has length Y. The distribution of Y is obtained and is related to previous work. When E is specialized to G or to ∂G, other known results are retrieved. The paper ends with a discussion of a conjecture relating the means of R, W and Y.
is to a random point in ∂G and has length Y. The distribution of Y is obtained and is related to previous work. When E is specialized to G or to ∂G, other known results are retrieved. The paper ends with a discussion of a conjecture relating the means of R, W and Y.
The authors acknowledge the support of the National Science and Engineering Research Council of Canada. Part of this work was completed at the Australian National University.