Article contents
Mean-square and almost-sure convergence of supercritical age-dependent branching processes
Published online by Cambridge University Press: 14 July 2016
Abstract
Letting Z(t) be the number of objects alive at time t in a general supercritical age-dependent branching process generated by a single ancestor born at time 0, one achieves (Theorem 1) mean-square convergence of Z(t)/E[Z(t)] provided 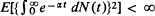 and
and 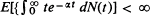 , where N(t) is the number of offspring of the initial ancestor born by time t and α is the (positive) Malthusian parameter defined by
, where N(t) is the number of offspring of the initial ancestor born by time t and α is the (positive) Malthusian parameter defined by 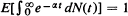 . If the stronger conditions that (Theorem 2)
. If the stronger conditions that (Theorem 2) 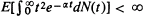 and
and  hold also, then the convergence is almost-sure. It is of interest that the embedded Galton-Watson process of successive generations need not have a finite mean for the conditions of the above theorems to hold. Similar results are obtained for the age-distribution as well.
hold also, then the convergence is almost-sure. It is of interest that the embedded Galton-Watson process of successive generations need not have a finite mean for the conditions of the above theorems to hold. Similar results are obtained for the age-distribution as well.
Keywords
Information
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1974
References
- 3
- Cited by

