Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gutiérrez, Cristina
and
Minuesa, Carmen
2020.
A Predator–Prey Two-Sex Branching Process.
Mathematics,
Vol. 8,
Issue. 9,
p.
1408.

 . It is proven that
. It is proven that 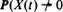 and
and 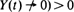 iff
iff