Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kolchin, V. F.
1977.
Branching processes, random trees, and a generalized scheme of arrangements of particles.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 21,
Issue. 5,
p.
386.
Kolchin, V. F.
1978.
Moment of degeneration of a branching process and height of a random tree.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 24,
Issue. 6,
p.
954.
Grimmett, G. R.
1980.
Random labelled trees and their branching networks.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 30,
Issue. 2,
p.
229.
Vatutin, V. A.
and
Zubkov, A. M.
1987.
Branching processes. I.
Journal of Soviet Mathematics,
Vol. 39,
Issue. 1,
p.
2431.
Gupta, Vijay K.
Mesa, Oscar J.
and
Waymire, E.
1990.
Tree-dependent extreme values: the exponential case.
Journal of Applied Probability,
Vol. 27,
Issue. 01,
p.
124.
Durrett, Rick
Kesten, Harry
and
Waymire, Ed
1991.
On weighted heights of random trees.
Journal of Theoretical Probability,
Vol. 4,
Issue. 1,
p.
223.
Waymire, Edward C.
1993.
New Directions in Time Series Analysis.
Vol. 46,
Issue. ,
p.
363.
Barndorff-Nielsen, Ole E.
1993.
Networks and Chaos — Statistical and Probabilistic Aspects.
p.
276.
Gutjahr, Walter
1993.
Expectation transfer between branching processes and random trees.
Random Structures & Algorithms,
Vol. 4,
Issue. 4,
p.
447.
Vatutin, V. A.
1994.
The Distribution of the Distance to the Root of the Minimal Subtree Containing All the Vertices of a Given Height.
Theory of Probability & Its Applications,
Vol. 38,
Issue. 2,
p.
330.
Kesten, Harry
1994.
The Dynkin Festschrift.
p.
153.
Gittenberger, Bernhard
1998.
Convergence of branching processes to the local time of a Bessel process.
Random Structures & Algorithms,
Vol. 13,
Issue. 3-4,
p.
423.
Devroye, Luc
1998.
Probabilistic Methods for Algorithmic Discrete Mathematics.
Vol. 16,
Issue. ,
p.
249.
Bennies, Jürgen
and
Kersting, Götz
2000.
A Random Walk Approach to Galton–Watson Trees.
Journal of Theoretical Probability,
Vol. 13,
Issue. 3,
p.
777.
Mylläri, T.
2002.
Limit distributions for the number of leaves in a random forest.
Advances in Applied Probability,
Vol. 34,
Issue. 4,
p.
904.
Geiger, Jochen
and
Kauffmann, Lars
2004.
The shape of large Galton‐Watson trees with possibly infinite variance.
Random Structures & Algorithms,
Vol. 25,
Issue. 3,
p.
311.
Drmota, Michael
2004.
Stochastic analysis of tree–like data structures.
Proceedings of the Royal Society of London. Series A: Mathematical, Physical and Engineering Sciences,
Vol. 460,
Issue. 2041,
p.
271.
Minami, Nariyuki
2005.
On the number of vertices with a given degree in a Galton-Watson tree.
Advances in Applied Probability,
Vol. 37,
Issue. 1,
p.
229.
Broutin, N.
Devroye, L.
McLeish, E.
and
de la Salle, M.
2008.
The height of increasing trees.
Random Structures & Algorithms,
Vol. 32,
Issue. 4,
p.
494.
Devroye, Luc
2009.
On exact simulation algorithms for some distributions related to Jacobi theta functions.
Statistics & Probability Letters,
Vol. 79,
Issue. 21,
p.
2251.

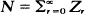 As
As 