Article contents
The convergence of a branching Brownian motion used as a model describing the spread of an epidemic
Published online by Cambridge University Press: 14 July 2016
Abstract
A spatial epidemic process where the individuals are located at positions in the Euclidean space R 2 is considered. The infective individuals, with an infection period that is exponentially distributed with parameter µ, move in R 2 according to a Brownian motion with a diffusion coefficient σ2. The susceptible individuals may also move. But we shall use the approximation that they remain unchanged in numbers and therefore assume that the averaged ‘density' of susceptibles per unit area is the same throughout space and time. The transition probability rate of infection of a susceptible in the infinitesimal element of area dy by an infective in dx is assumed to be a function h(x – y |) of the distance | x – y | between x and y. Then our process can be considered as a two-dimensional birth and death Brownian motion. Let  be the number of infective individuals in the set D at time t and
be the number of infective individuals in the set D at time t and 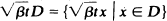 . The almost everywhere convergence of the random variables
. The almost everywhere convergence of the random variables 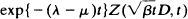 to a limit random variable W(D) is established.
to a limit random variable W(D) is established.
Information
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1980
References
- 4
- Cited by

