Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kovalenko, Igor N.
1994.
Rare events in queueing systems?A survey.
Queueing Systems,
Vol. 16-16,
Issue. 1-2,
p.
1.
Embrechts, P.
and
Klüppelberg, C.
1994.
Some Aspects of Insurance Mathematics.
Theory of Probability & Its Applications,
Vol. 38,
Issue. 2,
p.
262.
Novak, S. Yu.
1997.
On the Distribution of the Ratioof Sums of Random Variables.
Theory of Probability & Its Applications,
Vol. 41,
Issue. 3,
p.
479.
Diaz, Francisco J.
1999.
Identifying Tail Behavior by Means of Residual Quantile Functions.
Journal of Computational and Graphical Statistics,
Vol. 8,
Issue. 3,
p.
493.
Zeevi, Assaf
and
Glynn, Peter W.
2004.
Estimating tail decay for stationary sequences via extreme values.
Advances in Applied Probability,
Vol. 36,
Issue. 1,
p.
198.
Duffy, Ken R.
and
Meyn, Sean P.
2011.
Estimating Loynes’ exponent.
Queueing Systems,
Vol. 68,
Issue. 3-4,
p.
285.
Namkoong, Hongseok
Ma, Yuanzhe
and
Glynn, Peter W.
2025.
Minimax Optimal Estimation of Stability Under Distribution Shift.
Operations Research,
Schneble, Marc
and
Kauermann, Göran
2025.
Statistical modelling of on-street parking spot occupancy in smart cities.
Journal of the Royal Statistical Society Series C: Applied Statistics,
Vol. 74,
Issue. 4,
p.
1128.

 where
where 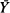 is the mean of the sample values overshooting
is the mean of the sample values overshooting 