Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Davis, Richard A.
and
Resnick, Sidney I.
1989.
Basic properties and prediction of max-ARMA processes.
Advances in Applied Probability,
Vol. 21,
Issue. 4,
p.
781.
Lewis, Peter A. W.
and
McKenzie, Ed
1991.
Minification processes and their transformations.
Journal of Applied Probability,
Vol. 28,
Issue. 01,
p.
45.
Littlejohn, R. P.
1992.
Discrete minification processes and reversibility.
Journal of Applied Probability,
Vol. 29,
Issue. 01,
p.
82.
Adke, S.R.
and
Balakrishna, N.
1992.
Estimation of the mean of some stationary markov sequences.
Communications in Statistics - Theory and Methods,
Vol. 21,
Issue. 1,
p.
137.
Littlejohn, R. P.
1994.
A reversibility relationship for two Markovian time series models by stationary exponential tailed distribution.
Journal of Applied Probability,
Vol. 31,
Issue. 02,
p.
575.
Adke, S. R.
and
Chandran, C.
1994.
Asymptotic distributions of extremes of extremal Markov sequences.
Journal of Applied Probability,
Vol. 31,
Issue. 01,
p.
256.
Rachev, Svetlozar T.
and
Samorodnitsky, Gennady
1995.
Limit laws for a stochastic process and random recursion arising in probabilistic modelling.
Advances in Applied Probability,
Vol. 27,
Issue. 01,
p.
185.
Kalamkar, V. A.
1995.
Minification processes with discrete marginals.
Journal of Applied Probability,
Vol. 32,
Issue. 03,
p.
692.
Littlejohn, R.P.
1996.
A reversibility relationship for two Markovian time series models with stationary geometric tailed distribution.
Stochastic Processes and their Applications,
Vol. 64,
Issue. 1,
p.
127.
Sreehari, M.
and
Kalamkar, V.A.
1997.
Modelling some stationary Markov processes and related characterizations.
Journal of Statistical Planning and Inference,
Vol. 63,
Issue. 2,
p.
363.
Balakrishna, N.
and
Jacob, T. M.
2003.
Parameter Estimation in Minification Processes.
Communications in Statistics - Theory and Methods,
Vol. 32,
Issue. 11,
p.
2139.
Lawrance, A. J.
2005.
Encyclopedia of Biostatistics.
2008.
Special Functions for Applied Scientists.
p.
247.
Lawrance, A. J.
2014.
Wiley StatsRef: Statistics Reference Online.
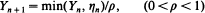 for {η n } a sequence of independent identically distributed (i.i.d.) random variables which are independent of {Yn }, and
for {η n } a sequence of independent identically distributed (i.i.d.) random variables which are independent of {Yn }, and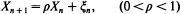 for {ξ n } a sequence of i.i.d. random variables which are independent of {Xn }, are mutually time-reversed if and only if their common marginal distribution is exponential, relating the exponential autoregressive process of Gaver and Lewis (1980) to the exponential minification process of Tavares (1980).
for {ξ n } a sequence of i.i.d. random variables which are independent of {Xn }, are mutually time-reversed if and only if their common marginal distribution is exponential, relating the exponential autoregressive process of Gaver and Lewis (1980) to the exponential minification process of Tavares (1980).
