Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kaplan, Norman
and
Pakes, A.G.
1974.
Supercritical age-dependent branching processes with immigration.
Stochastic Processes and their Applications,
Vol. 2,
Issue. 4,
p.
371.
Grey, D. R.
1976.
On possible rates of growth of age-dependent branching processes with immigration.
Journal of Applied Probability,
Vol. 13,
Issue. 01,
p.
138.
Parthasarathy, P.R.
and
Krishnamoorthy, A.
1977.
Compounding Bellman-Harris processes allowing immigration.
Mathematical Biosciences,
Vol. 37,
Issue. 1-2,
p.
105.
Vatutin, V. A.
and
Zubkov, A. M.
1987.
Branching processes. I.
Journal of Soviet Mathematics,
Vol. 39,
Issue. 1,
p.
2431.
Rahimov, I.
1995.
Branching Processes.
Vol. 99,
Issue. ,
p.
58.
Бутковский, Олег Александрович
and
Butkovskij, Oleg Aleksandrovich
2012.
Предельное поведение критического ветвящегося процесса с иммиграцией.
Математические заметки,
Vol. 92,
Issue. 5,
p.
670.
Butkovsky, O. A.
2012.
Limit behavior of a critical branching process with immigration.
Mathematical Notes,
Vol. 92,
Issue. 5-6,
p.
612.
SUN, HONGWEI
and
WU, QIANG
2013.
INDEFINITE KERNEL NETWORK WITH DEPENDENT SAMPLING.
Analysis and Applications,
Vol. 11,
Issue. 05,
p.
1350020.
Zhang, Meijian
and
Sun, Hongwei
2017.
Regression learning with non-identically and non-independently sampling.
International Journal of Wavelets, Multiresolution and Information Processing,
Vol. 15,
Issue. 01,
p.
1750007.

 . These particles evolve according to a one-dimensional age-dependent branching process with offspring p.g.f.
. These particles evolve according to a one-dimensional age-dependent branching process with offspring p.g.f. 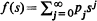 and life time distribution
and life time distribution  . Then it is shown that
. Then it is shown that 