No CrossRef data available.
Article contents
SUBMODULES OF COMMUTATIVE C*-ALGEBRAS
Published online by Cambridge University Press: 13 August 2013
Abstract
In this paper we generalise a result of Izuchi and Suárez (K. Izuchi and D. Suárez, Norm-closed invariant subspaces in L∞ and H∞, Glasgow Math. J. 46 (2004), 399–404) on the shift invariant subspaces of 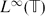 $L^\infty(\mathbb{T})$ to the non-commutative setting. Considering these subspaces as
$L^\infty(\mathbb{T})$ to the non-commutative setting. Considering these subspaces as 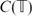 $C(\mathbb{T})$-modules contained in
$C(\mathbb{T})$-modules contained in 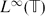 $L^\infty(\mathbb{T})$, we show that under some restrictions, a similar description can be given for the
$L^\infty(\mathbb{T})$, we show that under some restrictions, a similar description can be given for the 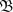 ${\mathfrak{B}}$-submodules of
${\mathfrak{B}}$-submodules of 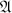 ${\mathfrak{A}}$, where
${\mathfrak{A}}$, where 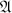 ${\mathfrak{A}}$ is a C*-algebra and
${\mathfrak{A}}$ is a C*-algebra and 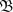 ${\mathfrak{B}}$ is a commutative C*-subalgebra of
${\mathfrak{B}}$ is a commutative C*-subalgebra of 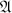 ${\mathfrak{A}}$. We use this to give a description of the
${\mathfrak{A}}$. We use this to give a description of the 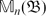 $\mathbb{M}_n({\mathfrak{B}})$-submodules of
$\mathbb{M}_n({\mathfrak{B}})$-submodules of 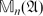 $\mathbb{M}_n({\mathfrak{A}})$.
$\mathbb{M}_n({\mathfrak{A}})$.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2013

