Article contents
SOME RESULTS OF THE 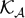 $\mathcal K_{\mathcal A}$-APPROXIMATION PROPERTY FOR BANACH SPACES
$\mathcal K_{\mathcal A}$-APPROXIMATION PROPERTY FOR BANACH SPACES
Published online by Cambridge University Press: 09 August 2018
Abstract
Given a Banach operator ideal 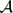 $\mathcal A$, we investigate the approximation property related to the ideal of
$\mathcal A$, we investigate the approximation property related to the ideal of 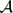 $\mathcal A$-compact operators,
$\mathcal A$-compact operators, 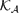 $\mathcal K_{\mathcal A}$-AP. We prove that a Banach space X has the
$\mathcal K_{\mathcal A}$-AP. We prove that a Banach space X has the 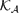 $\mathcal K_{\mathcal A}$-AP if and only if there exists a λ ≥ 1 such that for every Banach space Y and every R ∈
$\mathcal K_{\mathcal A}$-AP if and only if there exists a λ ≥ 1 such that for every Banach space Y and every R ∈ 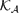 $\mathcal K_{\mathcal A}$(Y, X),
$\mathcal K_{\mathcal A}$(Y, X), $$\begin{equation}R \in \overline {\{SR : S \in \mathcal F(X, X), \|SR\|_{\mathcal K_{\mathcal A}} \leq \lambda \|R\|_{\mathcal K_{\mathcal A}}\}}^{\tau_{c}}.\end{equation}$$
$$\begin{equation}R \in \overline {\{SR : S \in \mathcal F(X, X), \|SR\|_{\mathcal K_{\mathcal A}} \leq \lambda \|R\|_{\mathcal K_{\mathcal A}}\}}^{\tau_{c}}.\end{equation}$$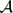 $\mathcal A$, we prove that a Banach space X has the
$\mathcal A$, we prove that a Banach space X has the 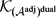 $\mathcal K_{(\mathcal A^{{\rm adj}})^{{\rm dual}}}$-AP if the dual space of X has the
$\mathcal K_{(\mathcal A^{{\rm adj}})^{{\rm dual}}}$-AP if the dual space of X has the 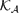 $\mathcal K_{\mathcal A}$-AP.
$\mathcal K_{\mathcal A}$-AP.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2018
References
REFERENCES
- 3
- Cited by

