Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sander, J. W.
1987.
A reciprocity formula for quadratic forms.
Monatshefte f�r Mathematik,
Vol. 104,
Issue. 2,
p.
125.
Yuan, Wang
1989.
On small zeros of quadratic forms over finite fields.
Journal of Number Theory,
Vol. 31,
Issue. 3,
p.
272.
Gérardin, Paul
and
Chung Yuan Lin
1989.
An identity involving quadratic characters.
Journal of Number Theory,
Vol. 33,
Issue. 3,
p.
356.
Cochrane, Todd
1989.
Small zeros of quadratic forms modulo p.
Journal of Number Theory,
Vol. 33,
Issue. 3,
p.
286.
Cochrane, Todd
1990.
Small zeros of quadratic congruences modulo
pq
.
Mathematika,
Vol. 37,
Issue. 2,
p.
261.
Cochrane, Todd
1990.
Analytic Number Theory.
p.
91.
Heath-Brown, D. R.
1991.
Small solutions of quadratic congruences, II.
Mathematika,
Vol. 38,
Issue. 2,
p.
264.
Cochrane, Todd
1991.
Small zeros of quadratic forms modulo p, III.
Journal of Number Theory,
Vol. 37,
Issue. 1,
p.
92.
Grant, David
1992.
Small solutions to a given quadratic form with a variable modulus.
Journal of Number Theory,
Vol. 42,
Issue. 1,
p.
57.
Yuan, Wang
1993.
On small zeros of quadratic forms over finite fields (II).
Acta Mathematica Sinica,
Vol. 9,
Issue. 4,
p.
382.
Cochrane, Todd
and
Zhiyong, Zheng
1998.
Small solutions of the congruencea 1 x 1 2 +a 2 x 2 2 +a 3 x 3 2 +a 4 x 4 2 ≡c(modp).
Acta Mathematica Sinica,
Vol. 14,
Issue. 2,
p.
175.
Hakami, A. H.
2015.
Small primitive zeros of quadratic forms mod $$p^m$$ p m.
The Ramanujan Journal,
Vol. 38,
Issue. 1,
p.
189.
Heath-Brown, D. R.
2016.
SMALL SOLUTIONS OF QUADRATIC CONGRUENCES, AND CHARACTER SUMS WITH BINARY QUADRATIC FORMS.
Mathematika,
Vol. 62,
Issue. 2,
p.
551.
Brüdern, Jörg
and
Dietmann, Rainer
2016.
Random congruences.
Indagationes Mathematicae,
Vol. 27,
Issue. 2,
p.
480.
Baier, Stephan
and
Haldar, Anup
2022.
Asymptotic behavior of small solutions of quadratic congruences in three variables modulo prime powers.
Research in Number Theory,
Vol. 8,
Issue. 3,

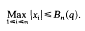
 gives the trivial lower bound
gives the trivial lower bound 