Article contents
ROOTS OF DEHN TWISTS ABOUT MULTICURVES
Published online by Cambridge University Press: 30 October 2017
Abstract
A multicurve 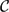 ${\mathcal{C}}$ in a closed orientable surface Sg of genus g is defined to be a finite collection of disjoint non-isotopic essential simple closed curves. A left-handed Dehn twist
${\mathcal{C}}$ in a closed orientable surface Sg of genus g is defined to be a finite collection of disjoint non-isotopic essential simple closed curves. A left-handed Dehn twist 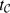 $t_{\mathcal{C}}$ about
$t_{\mathcal{C}}$ about 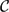 ${\mathcal{C}}$ is the product of left-handed Dehn twists about the individual curves in
${\mathcal{C}}$ is the product of left-handed Dehn twists about the individual curves in 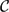 ${\mathcal{C}}$. In this paper, we derive necessary and sufficient conditions for the existence of a root of
${\mathcal{C}}$. In this paper, we derive necessary and sufficient conditions for the existence of a root of 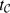 $t_{\mathcal{C}}$ in the mapping class group Mod(Sg). Using these conditions, we obtain combinatorial data that correspond to roots, and use it to determine upper bounds on the degree of a root. As an application of our theory, we classify all such roots up to conjugacy in Mod(S4). Finally, we establish that no such root can lie in the level m congruence subgroup of Mod(Sg), for m ≥ 3.
$t_{\mathcal{C}}$ in the mapping class group Mod(Sg). Using these conditions, we obtain combinatorial data that correspond to roots, and use it to determine upper bounds on the degree of a root. As an application of our theory, we classify all such roots up to conjugacy in Mod(S4). Finally, we establish that no such root can lie in the level m congruence subgroup of Mod(Sg), for m ≥ 3.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2017
References
REFERENCES
- 5
- Cited by

