No CrossRef data available.
Article contents
ON THE STANLEY DEPTH AND SIZE OF MONOMIAL IDEALS
Published online by Cambridge University Press: 14 March 2017
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let 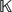 $\mathbb{K}$ be a field and S =
$\mathbb{K}$ be a field and S = 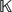 ${\mathbb{K}}$ [x 1, . . ., xn ] be the polynomial ring in n variables over the field
${\mathbb{K}}$ [x 1, . . ., xn ] be the polynomial ring in n variables over the field 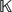 $\mathbb{K}$ . For every monomial ideal I ⊂ S, we provide a recursive formula to determine a lower bound for the Stanley depth of S/I. We use this formula to prove the inequality sdepth(S/I) ≥ size(I) for a particular class of monomial ideals.
$\mathbb{K}$ . For every monomial ideal I ⊂ S, we provide a recursive formula to determine a lower bound for the Stanley depth of S/I. We use this formula to prove the inequality sdepth(S/I) ≥ size(I) for a particular class of monomial ideals.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2017
References
REFERENCES
1.
Duval, A. M., Goeckner, B., Klivans, C. J. and Martin, J. L., A non-partitionable Cohen-Macaulay simplicial complex, Adv. Math. 299 (2016), 381–395.Google Scholar
2.
Herzog, J., A survey on Stanley depth, in Monomial ideals, computations and applications, Proceedings of MONICA 2011, Lecture Notes in Math., vol. 2083 (Bigatti, A., Giménez, P. and Sáenz-de-Cabezón, E., Editors) (Springer, Heidelberg, 2013) 3–45.CrossRefGoogle Scholar
3.
Herzog, J. and Hibi, T., Monomial ideals. Graduate Texts in Mathematics, 260. (Springer-Verlag, London, 2011).Google Scholar
4.
Herzog, J., Popescu, D. and Vladoiu, M., Stanley depth and size of a monomial ideal, Proc. Amer. Math. Soc.
140
(2) (2012), 493–504.CrossRefGoogle Scholar
5.
Ichim, B., Katthän, L. and Moyano-Fernández, J. J., The behavior of Stanley depth under polarization, J. Combin. Theory Ser. A
135 (2015), 332–347.CrossRefGoogle Scholar
6.
Lyubeznik, G., On the arithmetical rank of monomial ideals, J. Algebra
112
(1) (1988), 86–89.CrossRefGoogle Scholar
7.
Popescu, A., Special Stanley decompositions, Bull. Math. Soc. Sci. Math. Roumanie (N.S.)
53
(101) (2010), 361–373.Google Scholar
8.
Popescu, D., The Stanley conjecture on intersections of four monomial prime ideals, Comm. Algebra
41
(11) (2013), 4351–4362.Google Scholar
9.
Pournaki, M. R., Seyed Fakhari, S. A., Tousi, M. and Yassemi, S., What is . . . Stanley depth?
Notices Amer. Math. Soc.
56
(9) (2009), 1106–1108.Google Scholar
10.
Rauf, A., Stanley decompositions, pretty clean filtrations and reductions modulo regular elements, Bull. Math. Soc. Sci. Math. Roumanie (N.S.)
50
(98)(4) (2007), 347–354.Google Scholar
11.
Stanley, R. P., Linear Diophantine equations and local cohomology, Invent. Math.
68
(2) (1982), 175–193.CrossRefGoogle Scholar
12.
Tang, Z., Stanley depths of certain Stanley–Reisner rings, J. Algebra.
409 (2014), 430–443.Google Scholar

