Hostname: page-component-857557d7f7-9f75d Total loading time: 0 Render date: 2025-11-20T05:26:43.884Z Has data issue: false hasContentIssue false
  • English
  • Français

On Salié's sum

Published online by Cambridge University Press:  18 May 2009

L. J. Mordell
L. J. Mordell
Affiliation:
St. John's College, Cambridge
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let p be an odd prime and let f(x) be a complex-valued function such that f(x+p) = f(x) for all integers x. Write e(x) = exp(2πix/p), and define l/x by 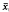, where 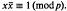We consider the sum

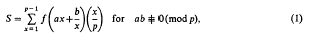
where 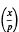is the Legendre symbol. The sum is zero if 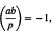as is clear on replacing x by bjax. Salié has found a result which can be written in the form

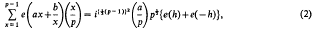
when h2 ≡ 4ab(modp).

Information

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 14 , Issue 1 , February 1973 , pp. 25 - 26
Copyright
Copyright © Glasgow Mathematical Journal Trust 1973

References

REFERENCES

1.Mordell, L. J., On some exponential sums related to Kloosterman sums, Ada Arithmetical to appear.Google Scholar
2.Williams, K. S., On Salié's sum, J. Number Theory 3 (1971), 316–317.CrossRefGoogle Scholar