No CrossRef data available.
Article contents
A NOTE ON THE GENUS OF GLOBAL FUNCTION FIELDS
Published online by Cambridge University Press: 26 February 2013
Abstract
To give a relatively elementary proof of the Brumer–Stark conjecture in a function field context involving no algebraic geometry beyond the Riemann–Roch theorem for curves, Hayes Compos. Math., vol. 55, 1985, pp. 209–239) defined a normalizing field 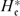 $H_\mathfrak{e}^*$ associated with a fixed sgn-normalized Drinfeld module and its extension field
$H_\mathfrak{e}^*$ associated with a fixed sgn-normalized Drinfeld module and its extension field 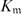 $K_\mathfrak{m}$, which is an analogue of cyclotomic function fields over a rational function field. We present explicitly in this note the formulae for the genus of the two fields and the maximal real subfield
$K_\mathfrak{m}$, which is an analogue of cyclotomic function fields over a rational function field. We present explicitly in this note the formulae for the genus of the two fields and the maximal real subfield 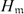 $H_\mathfrak{m}$ of
$H_\mathfrak{m}$ of 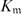 $K_\mathfrak{m}$. In some sense, our results can be regarded as generalizations of formulae for the genus of classical cyclotomic function fields obtained by Hayes Trans. Amer. Math. Soc., vol. 189, 1974, pp. 77–91) and Kida and Murabayashi (Tokyo J. Math., vol. 14(1), 1991, pp. 45–56).
$K_\mathfrak{m}$. In some sense, our results can be regarded as generalizations of formulae for the genus of classical cyclotomic function fields obtained by Hayes Trans. Amer. Math. Soc., vol. 189, 1974, pp. 77–91) and Kida and Murabayashi (Tokyo J. Math., vol. 14(1), 1991, pp. 45–56).
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2013

