Article contents
Hereditary semisimple classes
Published online by Cambridge University Press: 18 May 2009
Extract
It is well-known (see e.g. [1, p. 5]) that a class ℳ of (not necessarily associative) rings is the semisimple class for some radical class, relative to some universal class  if and only if it has the following properties:
if and only if it has the following properties:
(a)if ℳ, then every non-zero ideal I of Rhas a non-zero homomorphic image I/J∈ℳ.
(b) If R∈ but R∉ℳ, then R has a non-zero ideal I∈
but R∉ℳ, then R has a non-zero ideal I∈ , where
, where  ℳ = {K ∈
ℳ = {K ∈  | every non-zero K/H∉ℳ}. In fact
| every non-zero K/H∉ℳ}. In fact  ℳ is the radical class whose semisimple class is ℳ. On the other hand, if ℘ is a radical class, then ℐ℘ = {K∈
ℳ is the radical class whose semisimple class is ℳ. On the other hand, if ℘ is a radical class, then ℐ℘ = {K∈ / if I is a non-zero ideal of K, then I∉℘} is its semisimple class. If a class ℳ is hereditary (that is, when R∈ℳ, then all its ideals are in ℳ), it clearly satisfies (a), but there do exist non-hereditary semisimple classes (see [2]). The condition (satisfied in all associative or alternative classes) is that
/ if I is a non-zero ideal of K, then I∉℘} is its semisimple class. If a class ℳ is hereditary (that is, when R∈ℳ, then all its ideals are in ℳ), it clearly satisfies (a), but there do exist non-hereditary semisimple classes (see [2]). The condition (satisfied in all associative or alternative classes) is that 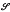 ℘ is hereditary for a radical class ℘ if and only if ℘(I) ⊆ ℘(R) for all ideals I of all rings R∈
℘ is hereditary for a radical class ℘ if and only if ℘(I) ⊆ ℘(R) for all ideals I of all rings R∈ [3, Lemma 2, p. 595].
[3, Lemma 2, p. 595].
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 1970
References
REFERENCES
- 6
- Cited by

