Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Nolla de Celis, Alvaro
2012.
Dihedral $G$-Hilb via representations of the McKay quiver.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 88,
Issue. 5,
Ishii, Akira
Nolla, Álvaro
and
Ueda, Kazushi
2024.
Dimer models and group actions.
Mathematische Zeitschrift,
Vol. 306,
Issue. 1,
Graffeo, Michele
2025.
Moduli spaces of ℤ/kℤ-constellations over 𝔸2.
Communications in Contemporary Mathematics,
Vol. 27,
Issue. 03,

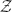 ⊂ℂ
⊂ℂ as vector spaces. These basis are the so-called
as vector spaces. These basis are the so-called 