Article contents
EXTREME POINTS FOR COMBINATORIAL BANACH SPACES
Published online by Cambridge University Press: 27 July 2018
Abstract
A norm ‖ċ‖ on c00 is called combinatorial if there is a regular family of finite subsets 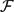 $\mathcal{F}$, so that
$\mathcal{F}$, so that 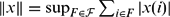 $\|x\|=\sup_{F \in \mathcal{F}} \sum_{i \in F} |x(i)|$. We prove the set of extreme points of the ball of a combinatorial Banach space is countable. This extends a theorem of Shura and Trautman. The second contribution of this article is to exhibit many new examples of extreme points for the unit ball of dual Tsirelson's original space and give an explicit construction of an uncountable collection of extreme points of the ball of Tsirelson's original space. We also prove some stability properties of the intermediate norms used to define Tsirelson's space and give a lower bound of the stabilization function for these intermediate norms.
$\|x\|=\sup_{F \in \mathcal{F}} \sum_{i \in F} |x(i)|$. We prove the set of extreme points of the ball of a combinatorial Banach space is countable. This extends a theorem of Shura and Trautman. The second contribution of this article is to exhibit many new examples of extreme points for the unit ball of dual Tsirelson's original space and give an explicit construction of an uncountable collection of extreme points of the ball of Tsirelson's original space. We also prove some stability properties of the intermediate norms used to define Tsirelson's space and give a lower bound of the stabilization function for these intermediate norms.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2018
Footnotes
Noah Duncan, Michael Holt and James Quigley were undergraduate students at Washington and Lee University when the main results of this paper were obtained.
References
REFERENCES
- 6
- Cited by

