No CrossRef data available.
Article contents
BGG CATEGORY FOR THE QUANTUM SCHRÖDINGER ALGEBRA
Published online by Cambridge University Press: 20 April 2020
Abstract
In 1996, a q-deformation of the universal enveloping algebra of the Schrödinger Lie algebra was introduced in Dobrev et al. [J. Phys. A 29 (1996) 5909–5918.]. This algebra is called the quantum Schrödinger algebra. In this paper, we study the Bernstein-Gelfand-Gelfand (BGG) category 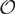 $\mathcal{O}$ for the quantum Schrödinger algebra
$\mathcal{O}$ for the quantum Schrödinger algebra 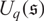 $U_q(\mathfrak{s})$, where q is a nonzero complex number which is not a root of unity. If the central charge
$U_q(\mathfrak{s})$, where q is a nonzero complex number which is not a root of unity. If the central charge 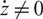 $\dot z\neq 0$, using the module
$\dot z\neq 0$, using the module 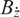 $B_{\dot z}$ over the quantum Weyl algebra
$B_{\dot z}$ over the quantum Weyl algebra 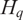 $H_q$, we show that there is an equivalence between the full subcategory
$H_q$, we show that there is an equivalence between the full subcategory 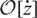 $\mathcal{O}[\dot Z]$ consisting of modules with the central charge
$\mathcal{O}[\dot Z]$ consisting of modules with the central charge  $\dot z$ and the BGG category
$\dot z$ and the BGG category 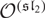 $\mathcal{O}^{(\mathfrak{sl}_2)}$ for the quantum group
$\mathcal{O}^{(\mathfrak{sl}_2)}$ for the quantum group 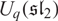 $U_q(\mathfrak{sl}_2)$. In the case that
$U_q(\mathfrak{sl}_2)$. In the case that 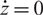 $\dot z = 0$, we study the subcategory
$\dot z = 0$, we study the subcategory 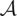 $\mathcal{A}$ consisting of finite dimensional
$\mathcal{A}$ consisting of finite dimensional 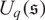 $U_q(\mathfrak{s})$-modules of type 1 with zero action of Z. We directly construct an equivalence functor from
$U_q(\mathfrak{s})$-modules of type 1 with zero action of Z. We directly construct an equivalence functor from 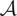 $\mathcal{A}$ to the category of finite dimensional representations of an infinite quiver with some quadratic relations. As a corollary, we show that the category of finite dimensional
$\mathcal{A}$ to the category of finite dimensional representations of an infinite quiver with some quadratic relations. As a corollary, we show that the category of finite dimensional 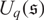 $U_q(\mathfrak{s})$-modules is wild.
$U_q(\mathfrak{s})$-modules is wild.
Information
- Type
- Research Article
- Information
- Copyright
- © The Author(s) 2020. Published by Cambridge University Press on behalf of Glasgow Mathematical Journal Trust

