Published online by Cambridge University Press: 14 February 2018
The Hajnal–Szemerédi theorem states that for any positive integer  $r$ and any multiple
$r$ and any multiple  $n$ of
$n$ of  $r$, if
$r$, if 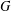 $G$ is a graph on
$G$ is a graph on  $n$ vertices and
$n$ vertices and 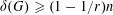 $\unicode[STIX]{x1D6FF}(G)\geqslant (1-1/r)n$, then
$\unicode[STIX]{x1D6FF}(G)\geqslant (1-1/r)n$, then  $G$ can be partitioned into
$G$ can be partitioned into 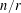 $n/r$ vertex-disjoint copies of the complete graph on
$n/r$ vertex-disjoint copies of the complete graph on  $r$ vertices. We prove a very general analogue of this result for directed graphs: for any positive integer
$r$ vertices. We prove a very general analogue of this result for directed graphs: for any positive integer  $r$ with
$r$ with 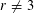 $r\neq 3$ and any sufficiently large multiple
$r\neq 3$ and any sufficiently large multiple  $n$ of
$n$ of  $r$, if
$r$, if 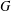 $G$ is a directed graph on
$G$ is a directed graph on  $n$ vertices and every vertex is incident to at least
$n$ vertices and every vertex is incident to at least  $2(1-1/r)n-1$ directed edges, then
$2(1-1/r)n-1$ directed edges, then  $G$ can be partitioned into
$G$ can be partitioned into 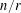 $n/r$ vertex-disjoint subgraphs of size
$n/r$ vertex-disjoint subgraphs of size  $r$ each of which contain every tournament on
$r$ each of which contain every tournament on  $r$ vertices (the case
$r$ vertices (the case 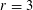 $r=3$ is different and was handled previously). In fact, this result is a consequence of a tiling result for standard multigraphs (that is multigraphs where there are at most two edges between any pair of vertices). A related Turán-type result is also proven.
$r=3$ is different and was handled previously). In fact, this result is a consequence of a tiling result for standard multigraphs (that is multigraphs where there are at most two edges between any pair of vertices). A related Turán-type result is also proven.