Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Temlyakov, Vladimir Nikolaevich
2015.
Конструктивные разреженные тригонометрические приближения и другие задачи для функций смешанной гладкости.
Математический сборник,
Vol. 206,
Issue. 11,
p.
131.
Temlyakov, V N
2015.
Constructive sparse trigonometric approximation and other problems for functions with mixed smoothness.
Sbornik: Mathematics,
Vol. 206,
Issue. 11,
p.
1628.
Temlyakov, V. N.
2015.
Greedy Approximation in Convex Optimization.
Constructive Approximation,
Vol. 41,
Issue. 2,
p.
269.
Bazarkhanov, D. B.
2016.
Nonlinear trigonometric approximations of multivariate function classes.
Proceedings of the Steklov Institute of Mathematics,
Vol. 293,
Issue. 1,
p.
2.
Temlyakov, Vladimir
2016.
New Trends in Applied Harmonic Analysis.
p.
107.
Shao, Chunfang
and
Ye, Peixin
2017.
Almost optimality of orthogonal super greedy algorithms for incoherent dictionaries.
International Journal of Wavelets, Multiresolution and Information Processing,
Vol. 15,
Issue. 04,
p.
1750029.
Cohen, Albert
Dahmen, Wolfgang
and
DeVore, Ronald
2017.
Orthogonal Matching Pursuit Under the Restricted Isometry Property.
Constructive Approximation,
Vol. 45,
Issue. 1,
p.
113.
Hilhorst, Pjotr L. J.
Quicken, Sjeng
van de Vosse, Frans N.
and
Huberts, Wouter
2024.
Efficient sensitivity analysis for biomechanical models with correlated inputs.
International Journal for Numerical Methods in Biomedical Engineering,
Vol. 40,
Issue. 2,
Albiac, Fernando
Ansorena, José L.
and
Temlyakov, Vladimir
2025.
Twenty-five years of greedy bases.
Journal of Approximation Theory,
Vol. 307,
Issue. ,
p.
106141.
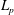 $L_p$,
$L_p$,  $2\le p<\infty $.
$2\le p<\infty $.