Article contents
REGULARIZATION OF NON-NORMAL MATRICES BY GAUSSIAN NOISE—THE BANDED TOEPLITZ AND TWISTED TOEPLITZ CASES
Published online by Cambridge University Press: 13 February 2019
Abstract
We consider the spectrum of additive, polynomially vanishing random perturbations of deterministic matrices, as follows. Let 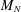 $M_{N}$ be a deterministic
$M_{N}$ be a deterministic  $N\times N$ matrix, and let
$N\times N$ matrix, and let 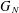 $G_{N}$ be a complex Ginibre matrix. We consider the matrix
$G_{N}$ be a complex Ginibre matrix. We consider the matrix  ${\mathcal{M}}_{N}=M_{N}+N^{-\unicode[STIX]{x1D6FE}}G_{N}$, where
${\mathcal{M}}_{N}=M_{N}+N^{-\unicode[STIX]{x1D6FE}}G_{N}$, where 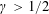 $\unicode[STIX]{x1D6FE}>1/2$. With
$\unicode[STIX]{x1D6FE}>1/2$. With 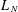 $L_{N}$ the empirical measure of eigenvalues of
$L_{N}$ the empirical measure of eigenvalues of  ${\mathcal{M}}_{N}$, we provide a general deterministic equivalence theorem that ties
${\mathcal{M}}_{N}$, we provide a general deterministic equivalence theorem that ties 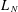 $L_{N}$ to the singular values of
$L_{N}$ to the singular values of 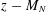 $z-M_{N}$, with
$z-M_{N}$, with  $z\in \mathbb{C}$. We then compute the limit of
$z\in \mathbb{C}$. We then compute the limit of 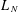 $L_{N}$ when
$L_{N}$ when 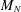 $M_{N}$ is an upper-triangular Toeplitz matrix of finite symbol: if
$M_{N}$ is an upper-triangular Toeplitz matrix of finite symbol: if  $M_{N}=\sum _{i=0}^{\mathfrak{d}}a_{i}J^{i}$ where
$M_{N}=\sum _{i=0}^{\mathfrak{d}}a_{i}J^{i}$ where 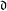 $\mathfrak{d}$ is fixed,
$\mathfrak{d}$ is fixed, 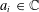 $a_{i}\in \mathbb{C}$ are deterministic scalars and
$a_{i}\in \mathbb{C}$ are deterministic scalars and 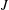 $J$ is the nilpotent matrix
$J$ is the nilpotent matrix  $J(i,j)=\mathbf{1}_{j=i+1}$, then
$J(i,j)=\mathbf{1}_{j=i+1}$, then 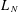 $L_{N}$ converges, as
$L_{N}$ converges, as 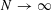 $N\rightarrow \infty$, to the law of
$N\rightarrow \infty$, to the law of 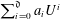 $\sum _{i=0}^{\mathfrak{d}}a_{i}U^{i}$ where
$\sum _{i=0}^{\mathfrak{d}}a_{i}U^{i}$ where 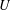 $U$ is a uniform random variable on the unit circle in the complex plane. We also consider the case of slowly varying diagonals (twisted Toeplitz matrices), and, when
$U$ is a uniform random variable on the unit circle in the complex plane. We also consider the case of slowly varying diagonals (twisted Toeplitz matrices), and, when  $\mathfrak{d}=1$, also of independent and identically distributed entries on the diagonals in
$\mathfrak{d}=1$, also of independent and identically distributed entries on the diagonals in 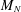 $M_{N}$.
$M_{N}$.
MSC classification
Information
- Type
- Research Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © The Author(s) 2019
References
- 10
- Cited by

