Article contents
QUASIRANDOM GROUP ACTIONS
Published online by Cambridge University Press: 30 August 2016
Abstract
Let 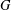 $G$ be a finite group acting transitively on a set
$G$ be a finite group acting transitively on a set  $\unicode[STIX]{x1D6FA}$ . We study what it means for this action to be quasirandom, thereby generalizing Gowers’ study of quasirandomness in groups. We connect this notion of quasirandomness to an upper bound for the convolution of functions associated with the action of
$\unicode[STIX]{x1D6FA}$ . We study what it means for this action to be quasirandom, thereby generalizing Gowers’ study of quasirandomness in groups. We connect this notion of quasirandomness to an upper bound for the convolution of functions associated with the action of 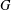 $G$ on
$G$ on 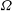 $\unicode[STIX]{x1D6FA}$ . This convolution bound allows us to give sufficient conditions such that sets
$\unicode[STIX]{x1D6FA}$ . This convolution bound allows us to give sufficient conditions such that sets 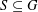 $S\subseteq G$ and
$S\subseteq G$ and 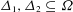 $\unicode[STIX]{x1D6E5}_{1},\unicode[STIX]{x1D6E5}_{2}\subseteq \unicode[STIX]{x1D6FA}$ contain elements
$\unicode[STIX]{x1D6E5}_{1},\unicode[STIX]{x1D6E5}_{2}\subseteq \unicode[STIX]{x1D6FA}$ contain elements 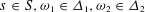 $s\in S,\unicode[STIX]{x1D714}_{1}\in \unicode[STIX]{x1D6E5}_{1},\unicode[STIX]{x1D714}_{2}\in \unicode[STIX]{x1D6E5}_{2}$ such that
$s\in S,\unicode[STIX]{x1D714}_{1}\in \unicode[STIX]{x1D6E5}_{1},\unicode[STIX]{x1D714}_{2}\in \unicode[STIX]{x1D6E5}_{2}$ such that 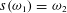 $s(\unicode[STIX]{x1D714}_{1})=\unicode[STIX]{x1D714}_{2}$ . Other consequences include an analogue of ‘the Gowers trick’ of Nikolov and Pyber for general group actions, a sum-product type theorem for large subsets of a finite field, as well as applications to expanders and to the study of the diameter and width of a finite simple group.
$s(\unicode[STIX]{x1D714}_{1})=\unicode[STIX]{x1D714}_{2}$ . Other consequences include an analogue of ‘the Gowers trick’ of Nikolov and Pyber for general group actions, a sum-product type theorem for large subsets of a finite field, as well as applications to expanders and to the study of the diameter and width of a finite simple group.
Information
- Type
- Research Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © The Author 2016
References
- 4
- Cited by


