Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bernier, Joackim
Feola, Roberto
Grébert, Benoît
and
Iandoli, Felice
2021.
Long-Time Existence for Semi-linear Beam Equations on Irrational Tori.
Journal of Dynamics and Differential Equations,
Vol. 33,
Issue. 3,
p.
1363.
Zhang, Qidi
and
Zheng, Lvsi
2021.
A Note on Lower Bound Lifespan Estimates for Semi-linear Wave/Klein–Gordon Equations Associated with the Harmonic Oscillator.
Bulletin of the Iranian Mathematical Society,
Vol. 47,
Issue. S1,
p.
171.
Bao, Weizhu
Feng, Yue
and
Su, Chunmei
2021.
Uniform error bounds of time-splitting spectral methods for the long-time dynamics of the nonlinear Klein–Gordon equation with weak nonlinearity.
Mathematics of Computation,
Vol. 91,
Issue. 334,
p.
811.
Cong, Hongzi
Mi, Lufang
and
Shi, Yunfeng
2022.
Super-exponential stability estimate for the nonlinear Schrödinger equation.
Journal of Functional Analysis,
Vol. 283,
Issue. 12,
p.
109682.
Faou, Erwan
2022.
Resonances and genericity in Birkhoff normal forms.
Séminaire Laurent Schwartz — EDP et applications,
p.
1.
Bao, Weizhu
Cai, Yongyong
and
Feng, Yue
2022.
Improved Uniform Error Bounds on Time-Splitting Methods for Long-Time Dynamics of the Nonlinear Klein--Gordon Equation with Weak Nonlinearity.
SIAM Journal on Numerical Analysis,
Vol. 60,
Issue. 4,
p.
1962.
Bernier, Joackim
and
Grébert, Benoît
2022.
Birkhoff normal forms for Hamiltonian PDEs in their energy space.
Journal de l’École polytechnique — Mathématiques,
Vol. 9,
Issue. ,
p.
681.
Cong, Hongzi
Mi, Lufang
Wu, Xiaoqing
and
Zhang, Qidi
2022.
Exponential stability estimate for the derivative nonlinear Schrödinger equation*
.
Nonlinearity,
Vol. 35,
Issue. 5,
p.
2385.
Brun, Pierre
2023.
Partial Normal Form for the Semilinear Klein–Gordon Equation with Quadratic Potentials and Algebraic Non-resonant Masses.
Journal of Dynamics and Differential Equations,
Vol. 35,
Issue. 3,
p.
2641.
Feola, Roberto
Grébert, Benoît
and
Iandoli, Felice
2023.
Long time solutions for quasilinear Hamiltonian
perturbations of Schrödinger and Klein–Gordon equations on tori.
Analysis & PDE,
Vol. 16,
Issue. 5,
p.
1133.
Bambusi, D.
Feola, R.
and
Montalto, R.
2024.
Almost Global Existence for Some Hamiltonian PDEs with Small Cauchy Data on General Tori.
Communications in Mathematical Physics,
Vol. 405,
Issue. 1,
Liu, Jianjun
and
Xiang, Duohui
2024.
Exact global control of small divisors in rational normal form
*
.
Nonlinearity,
Vol. 37,
Issue. 7,
p.
075020.
Cong, Hongzi
Shi, Yunfeng
and
Wu, Xiaoqing
2024.
Long-Time Anderson Localization for the Nonlinear Random Schrödinger Equation on $${\mathbb {Z}}^d$$.
Journal of Statistical Physics,
Vol. 191,
Issue. 9,
Liu, Jianjun
and
Xiang, Duohui
2024.
Long time stability for the derivative nonlinear Schrödinger equation.
Journal of Mathematical Analysis and Applications,
Vol. 537,
Issue. 2,
p.
128394.
Faou, Erwan
and
Grébert, Benoît
2025.
Discrete Pseudo-differential Operators and Applications to Numerical Schemes.
Foundations of Computational Mathematics,
Vol. 25,
Issue. 2,
p.
587.
Bernier, Joackim
and
Camps, Nicolas
2025.
Long time stability for cubic nonlinear Schrödinger equations on non-rectangular flat tori.
Journal de l’École polytechnique — Mathématiques,
Vol. 12,
Issue. ,
p.
713.
Cong, Hongzi
Ding, Wanran
Li, Siming
and
Wang, Peizhen
2025.
Sub-exponentially long timescale stability for nonlinear Klein–Gordon equation with potential.
Journal of Mathematical Physics,
Vol. 66,
Issue. 1,
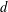 $d$-DIMENSIONAL TORUS
$d$-DIMENSIONAL TORUS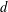 $d$-dimensional torus
$d$-dimensional torus 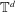 $\mathbb{T}^{d}$ with a smooth nonlinearity of order at least 2 at the origin. We prove that, for almost any mass, small and smooth solutions of high Sobolev indices are stable up to arbitrary long times with respect to the size of the initial data. To prove this result, we use a normal form transformation decomposing the dynamics into low and high frequencies with weak interactions. While the low part of the dynamics can be put under classical Birkhoff normal form, the high modes evolve according to a time-dependent linear Hamiltonian system. We then control the global dynamics by using polynomial growth estimates for high modes and the preservation of Sobolev norms for the low modes. Our general strategy applies to any semilinear Hamiltonian Partial Differential Equations (PDEs) whose linear frequencies satisfy a very general nonresonance condition. The (NLW) equation on
$\mathbb{T}^{d}$ with a smooth nonlinearity of order at least 2 at the origin. We prove that, for almost any mass, small and smooth solutions of high Sobolev indices are stable up to arbitrary long times with respect to the size of the initial data. To prove this result, we use a normal form transformation decomposing the dynamics into low and high frequencies with weak interactions. While the low part of the dynamics can be put under classical Birkhoff normal form, the high modes evolve according to a time-dependent linear Hamiltonian system. We then control the global dynamics by using polynomial growth estimates for high modes and the preservation of Sobolev norms for the low modes. Our general strategy applies to any semilinear Hamiltonian Partial Differential Equations (PDEs) whose linear frequencies satisfy a very general nonresonance condition. The (NLW) equation on 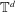 $\mathbb{T}^{d}$ is a good example since the standard Birkhoff normal form applies only when
$\mathbb{T}^{d}$ is a good example since the standard Birkhoff normal form applies only when  $d=1$ while our strategy applies in any dimension.
$d=1$ while our strategy applies in any dimension.
