Article contents
Linear evolution equations on the half-line with dynamic boundary conditions
Published online by Cambridge University Press: 03 May 2021
Abstract
The classical half-line Robin problem for the heat equation may be solved via a spatial Fourier transform method. In this work, we study the problem in which the static Robin condition 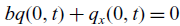 $$bq(0,t) + {q_x}(0,t) = 0$$ is replaced with a dynamic Robin condition;
$$bq(0,t) + {q_x}(0,t) = 0$$ is replaced with a dynamic Robin condition; 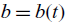 $$b = b(t)$$ is allowed to vary in time. Applications include convective heating by a corrosive liquid. We present a solution representation and justify its validity, via an extension of the Fokas transform method. We show how to reduce the problem to a variable coefficient fractional linear ordinary differential equation for the Dirichlet boundary value. We implement the fractional Frobenius method to solve this equation and justify that the error in the approximate solution of the original problem converges appropriately. We also demonstrate an argument for existence and unicity of solutions to the original dynamic Robin problem for the heat equation. Finally, we extend these results to linear evolution equations of arbitrary spatial order on the half-line, with arbitrary linear dynamic boundary conditions.
$$b = b(t)$$ is allowed to vary in time. Applications include convective heating by a corrosive liquid. We present a solution representation and justify its validity, via an extension of the Fokas transform method. We show how to reduce the problem to a variable coefficient fractional linear ordinary differential equation for the Dirichlet boundary value. We implement the fractional Frobenius method to solve this equation and justify that the error in the approximate solution of the original problem converges appropriately. We also demonstrate an argument for existence and unicity of solutions to the original dynamic Robin problem for the heat equation. Finally, we extend these results to linear evolution equations of arbitrary spatial order on the half-line, with arbitrary linear dynamic boundary conditions.
Keywords
Information
- Type
- Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
- 6
- Cited by


