Published online by Cambridge University Press: 19 June 2007
Let X1,...,Xn1 be arandom sample from a population with mean µ 1 and variance $\sigma_1^2$  , and X1,...,Xn1 be a random sample fromanother population with mean µ 2 and variance $\sigma_2^2$
, and X1,...,Xn1 be a random sample fromanother population with mean µ 2 and variance $\sigma_2^2$ 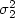 independent of{Xi,1 ≤ i ≤ n1 }.Consider the twosample t-statistic $ T={{\bar X-\bar Y-(\mu_1-\mu_2)} \over\sqrt{s_1^2/n_1+s_2^2/n_2}}$
independent of{Xi,1 ≤ i ≤ n1 }.Consider the twosample t-statistic $ T={{\bar X-\bar Y-(\mu_1-\mu_2)} \over\sqrt{s_1^2/n_1+s_2^2/n_2}}$  . This paper shows thatln P(T ≥ x) ~ -x²/2 for any x := x(n1,n2) satisfying x → ∞, x = o(n1 + n2)1/2 as n1,n2 → ∞ provided 0 < c1 ≤ n1/n2 ≤ c2 < ∞. If, inaddition, E|X1|3 < ∞, E|Y1|3 < ∞, then $\frac{P(T \geq x)}{1-\Phi(x)} \to 1 $
. This paper shows thatln P(T ≥ x) ~ -x²/2 for any x := x(n1,n2) satisfying x → ∞, x = o(n1 + n2)1/2 as n1,n2 → ∞ provided 0 < c1 ≤ n1/n2 ≤ c2 < ∞. If, inaddition, E|X1|3 < ∞, E|Y1|3 < ∞, then $\frac{P(T \geq x)}{1-\Phi(x)} \to 1 $  holds uniformly in x ∈ (O,o((n1 + n2)1/6))
holds uniformly in x ∈ (O,o((n1 + n2)1/6))