Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Papaspiliopoulos, Omiros
Roberts, Gareth O.
and
Taylor, Kasia B.
2016.
Exact sampling of diffusions with a discontinuity in the drift.
Advances in Applied Probability,
Vol. 48,
Issue. A,
p.
249.
Leobacher, Gunther
and
Szölgyenyi, Michaela
2016.
A numerical method for SDEs with discontinuous drift.
BIT Numerical Mathematics,
Vol. 56,
Issue. 1,
p.
151.
Leobacher, Gunther
and
Szölgyenyi, Michaela
2017.
A strong order $1/2$ method for multidimensional SDEs with discontinuous drift.
The Annals of Applied Probability,
Vol. 27,
Issue. 4,
Kohatsu-Higa, Arturo
Lejay, Antoine
and
Yasuda, Kazuhiro
2017.
Weak rate of convergence of the Euler–Maruyama scheme for stochastic differential equations with non-regular drift.
Journal of Computational and Applied Mathematics,
Vol. 326,
Issue. ,
p.
138.
Dereudre, David
Mazzonetto, Sara
and
Roelly, Sylvie
2017.
Exact Simulation of Brownian Diffusions with Drift Admitting Jumps.
SIAM Journal on Scientific Computing,
Vol. 39,
Issue. 3,
p.
A711.
Alfonsi, Aurélien
Hayashi, Masafumi
and
Kohatsu-Higa, Arturo
2017.
Modern Problems of Stochastic Analysis and Statistics.
Vol. 208,
Issue. ,
p.
43.
Ngo, Hoang-Long
and
Taguchi, Dai
2018.
Approximation for non-smooth functionals of stochastic differential equations with irregular drift.
Journal of Mathematical Analysis and Applications,
Vol. 457,
Issue. 1,
p.
361.
Chen, Yaming
and
Deng, Xiaogang
2018.
Numerical solutions of Fokker-Planck equations with drift-admitting jumps.
Physical Review E,
Vol. 98,
Issue. 3,
Frikha, Noufel
2018.
On the weak approximation of a skew diffusion by an Euler-type scheme.
Bernoulli,
Vol. 24,
Issue. 3,
Leobacher, Gunther
and
Szölgyenyi, Michaela
2018.
Convergence of the Euler–Maruyama method for multidimensional SDEs with discontinuous drift and degenerate diffusion coefficient.
Numerische Mathematik,
Vol. 138,
Issue. 1,
p.
219.
Neuenkirch, Andreas
Szölgyenyi, Michaela
and
Szpruch, Lukasz
2019.
An Adaptive Euler--Maruyama Scheme for Stochastic Differential Equations with Discontinuous Drift and its Convergence Analysis.
SIAM Journal on Numerical Analysis,
Vol. 57,
Issue. 1,
p.
378.
Pigato, Paolo
2019.
Extreme at-the-money skew in a local volatility model.
Finance and Stochastics,
Vol. 23,
Issue. 4,
p.
827.
Étoré, Pierre
and
Martinez, Miguel
2021.
A transformed stochastic Euler scheme for multidimensional transmission PDE.
Journal of Computational and Applied Mathematics,
Vol. 394,
Issue. ,
p.
113551.
Bencheikh, Oumaima
and
Jourdain, Benjamin
2022.
Convergence in Total Variation of the Euler--Maruyama Scheme Applied to Diffusion Processes with Measurable Drift Coefficient and Additive Noise.
SIAM Journal on Numerical Analysis,
Vol. 60,
Issue. 4,
p.
1701.
Kohatsu-Higa, Arturo
and
Tanaka, Akihiro
2024.
Weak Approximation for a Black-Scholes Type Regime Switching Model.
Applied Mathematical Finance,
Vol. 31,
Issue. 1,
p.
1.
Jourdain, Benjamin
and
Menozzi, Stéphane
2024.
Convergence rate of the Euler–Maruyama scheme applied to diffusion processes with Lq−Lρ drift coefficient and additive noise.
The Annals of Applied Probability,
Vol. 34,
Issue. 1B,
 dXt=dWt+b̅(Xt)dt, X0=x,
dXt=dWt+b̅(Xt)dt, X0=x,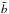 b̅ is a smooth real function except at point0 where \hbox{$\bar{b}(0+)\neq \bar{b}(0-)$}
b̅ is a smooth real function except at point0 where \hbox{$\bar{b}(0+)\neq \bar{b}(0-)$}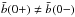 b̅(0 + ) ≠b̅(0 −). The main idea is to sample an exact skeleton ofX using analgorithm deduced from the convergence of the solutions of the skew perturbed equation(2)
b̅(0 + ) ≠b̅(0 −). The main idea is to sample an exact skeleton ofX using analgorithm deduced from the convergence of the solutions of the skew perturbed equation(2) dXtβ=dWt+b̅(Xtβ)dt+βdLt0(Xβ), X0=x
dXtβ=dWt+b̅(Xtβ)dt+βdLt0(Xβ), X0=x