Published online by Cambridge University Press: 15 August 2002
In the case of an elastic strip we exhibit two properties ofdispersion curves λn,n ≥ 1, that were not pointed outpreviously. We show cases where λ'n(0) = λ''n(0) = λ'''n(0) = 0 and we point out that these curves are not automatically monotoneous on ${\mathbb{R}}_{+}$ 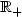 . The non monotonicity was an open question (see [2],for example) and, for the first time, we give a rigourous answer. Recall thecharacteristic property of the dispersion curves: {λn(p);n ≥ 1} isthe set of eigenvalues of A p , counted with their multiplicity. Theoperators Ap , $p\in{\mathbb{R}}$
. The non monotonicity was an open question (see [2],for example) and, for the first time, we give a rigourous answer. Recall thecharacteristic property of the dispersion curves: {λn(p);n ≥ 1} isthe set of eigenvalues of A p , counted with their multiplicity. Theoperators Ap , $p\in{\mathbb{R}}$  , are the reduced operators deduced from the elasticoperator A using a partial Fourier transform. The second goal of this article is the introduction of a dispersion relationD(p,λ) = 0 in a general framework, and not only for a homogeneous situation(in this last case the relation is explicit). Recall that a dispersionrelation isan implicit equation the solutions of which are eigenvalues of A p . The mainproperty of the function D that we build is the following one: themultiplicity of an eigenvalue λ of A p is equal to the multiplicity ithas as a root of D(p,λ) = 0. We give also some applications.
, are the reduced operators deduced from the elasticoperator A using a partial Fourier transform. The second goal of this article is the introduction of a dispersion relationD(p,λ) = 0 in a general framework, and not only for a homogeneous situation(in this last case the relation is explicit). Recall that a dispersionrelation isan implicit equation the solutions of which are eigenvalues of A p . The mainproperty of the function D that we build is the following one: themultiplicity of an eigenvalue λ of A p is equal to the multiplicity ithas as a root of D(p,λ) = 0. We give also some applications.