Published online by Cambridge University Press: 15 August 2004
In this paper, we consider a multi-lithology diffusion model used in stratigraphic modelling to simulate large scale transport processes of sediments described as a mixture of L lithologies. This model is a simplified one for which the surficial fluxes are proportional to the slope of the topography and to a lithology fraction with unitary diffusion coefficients.The main unknowns of the system are the sediment thickness h, the L surface concentrations $c_i^s$ 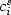 in lithology i of the sediments at the top of the basin, and the L concentrations c i in lithology i of the sediments inside the basin. For this simplified model, the sediment thickness decouples from the other unknowns and satisfies a linear parabolic equation. The remaining equations account for the mass conservation of the lithologies, and couple, for each lithology, a first order linear equation for $c_i^s$
in lithology i of the sediments at the top of the basin, and the L concentrations c i in lithology i of the sediments inside the basin. For this simplified model, the sediment thickness decouples from the other unknowns and satisfies a linear parabolic equation. The remaining equations account for the mass conservation of the lithologies, and couple, for each lithology, a first order linear equation for $c_i^s$ 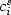 with a linear advection equation for c i for which $c_i^s$
with a linear advection equation for c i for which $c_i^s$ 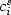 appears as an input boundary condition. For this coupled system, a weak formulation is introduced which is shown to have a unique solution. An implicit finite volume scheme is derived for which we show stability estimates and the convergence to the weak solution of the problem.
appears as an input boundary condition. For this coupled system, a weak formulation is introduced which is shown to have a unique solution. An implicit finite volume scheme is derived for which we show stability estimates and the convergence to the weak solution of the problem.