Published online by Cambridge University Press: 15 September 2002
In this work, we analyze hierarchic hp-finite element discretizations of the full, three-dimensional plate problem. Based on two-scale asymptotic expansion of the three-dimensional solution, we give specific mesh design principles for the hp-FEM which allow to resolve the three-dimensional boundary layer profiles at robust, exponential rate.We prove that, as the plate half-thickness ε tends to zero, the hp-discretization is consistent with the three-dimensional solution to any power of ε in the energy norm for the degree $p={\cal O}(\left|{\log \varepsilon}\right|)$ 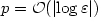 and with ${\cal O}({p^4})$
and with ${\cal O}({p^4})$ 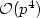 degrees of freedom.
degrees of freedom.