Hostname: page-component-cb9f654ff-d5ftd Total loading time: 0 Render date: 2025-08-24T17:04:05.332Z Has data issue: false hasContentIssue false
  • English
  • Français

Convergence analysis for an exponentially fittedFinite Volume Method

Published online by Cambridge University Press:  15 April 2002

Reiner Vanselow
Reiner Vanselow*
Affiliation:
Dresden University of Technology, Department of Mathematics, 01062 Dresden, Germany. (vanselow@math.tu-dresden.de)
Get access

Abstract

The paper is devoted to the convergence analysis of a well-knowncell-centered Finite Volume Method (FVM) for aconvection-diffusion problem in $\mathbb{R}^2$ 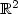. This FVM is based on Voronoi boxes andexponential fitting. To prove the convergence of the FVM, we usea new nonconforming Petrov-Galerkin Finite Element Method (FEM)for which the system of linear equations coincides completely withthat of the FVM. Thus, by proving convergence properties of theFEM we obtain similar ones for the FVM. For the error estimationof the FEM well-known statements have to be modified.

Keywords

Convection-diffusion problemcell-centered finite volume methodVoronoi boxesexponential fittingconvergence analysisnonconforming finite element method.

Information

Type
Research Article
Information
ESAIM: Mathematical Modelling and Numerical Analysis , Volume 34 , Issue 6 , November 2000 , pp. 1165 - 1188
Copyright
© EDP Sciences, SMAI, 2000

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Angermann, L., Error Estimate for the Finite-Element Solution of an Elliptic Singularly Perturbed Problem. IMA J. Numer. Anal. 15 (1995) 161-196. CrossRef
Bank, R.E., Bürgler, J.F., Fichtner, W. and Smith, R.K., Some Upwinding Techniques for Finite Element Approximations of Convection-Diffusion Equations. Numer. Math. 58 (1990) 185-202. CrossRef
Bank, R.E., Coughran, W.M. Jr. and Cowsar, L.C., The Finite Volume Scharfetter-Gummel Method for Steady Convection Diffusion Equations. Comput. Visual Sci. 1 (1998) 123-136. CrossRef
Baranger, J., Maitre, J.-F. and Oudin, F., Connection between Finite Volume and Mixed Finite Element Methods. RAIRO Modél. Math. Anal. Numér. 30 (1996) 445-465. CrossRef
D. Braess, Finite Elemente. Springer, Berlin (1992).
P.G. Ciarlet, Basic Error Estimates for Elliptic Problems, in Handbook of Numerical Analysis, Vol. II, Part 1, P.G. Ciarlet and J.L. Lions Eds., Elsevier, Amsterdam (1991) 17-351.
Eymard, R., Gallouet, T. and Herbin, R., Convergence of Finite Volume Schemes for Semilinear Convection Diffusion Equations. Numer. Math. 1 (1999) 1-26.
Gatti, E., Micheletti, S. and Sacco, R., New Galerkin Framework, A for the Drift-Diffusion Equation in Semiconductors. East-West J. Numer. Math. 6 (1998) 101-135.
B. Heinrich, Finite Difference Methods on Irregular Networks. A Generalized Approach to Second Order Problems. Akademie, Berlin (1987).
Herbin, R., Error Estimate, An for a Finite Volume Scheme for a Diffusion-Convection Problem on a Triangular Mesh. Numer. Methods Partial Differential Equations 11 (1995) 165-173. CrossRef
R.D. Lazarov and I.D. Mishev, Finite Volume Methods for Reaction-Diffusion Problems, in Finite Volumes for Complex Applications, F. Benkhaldoun and R. Vilsmeier Eds., Hermes, Paris (1996) 231-240.
Miller, J.J.H. and Wang, S., New Non-Conforming Petrov-Galerkin Finite El, Aement Method with Triangular Elements for an Advection-Diffusion Problem. IMA J. Numer. Anal. 14 (1994) 257-276. CrossRef
I.D. Mishev, Finite Volume and Finite Volume Element Methods for Nonsymmetric Problems. Ph.D. thesis, Texas A&M University (1996).
K.W. Morton, Numerical Solution of Convection-Diffusion Problems. Chapman and Hall, London (1996).
Morton, K.W., Stynes, M. and Süli, E., Analysis of a Cell-Vertex Finite Volume Method for Convection-Diffusion Problems. Math. Comp. 66 (1997) 1369-1406. CrossRef
H.G. Roos, M. Stynes and L. Tobiska, Numerical Methods for Singularly Perturbed Differential Equations. Springer, London (1996).
Sacco, R. and Stynes, M., Finite Element Methods for Convection-Diffusion Problems Using Exponential Splines on Triangles. Comput. Math. Appl. 35 (1998) 35-45. CrossRef
Sacco, R., Gatti, E. and Gotusso, L., Nonconforming Exponentially Fitted Finite El, Aement Method for Two-Dimensional Drift-Diffusion Models in Semiconductors. Numer. Methods Partial Differential Equations 15 (1999) 133-150. 3.0.CO;2-N>CrossRef
H.-P. Scheffler and R. Vanselow, Convergence Analysis of a Cell-Centered FVM, in Finite Volumes for Complex Applications II, R. Vilsmeier, F. Benkhaldoun and D. Hänel Eds., Hermes, Paris (1999) 181-188.
L.L. Schumaker, Spline Functions: Basic Theory. Wiley, New York (1981).
S. Selberherr, Analysis and Simulation of Semiconductor Devices. Springer, Wien (1984).
G. Strang, Variational Crimes in the Finite Element Method, in The Mathematical Foundations of the Finite Element Method with Applications to Partial Differential Equations, A.K. Aziz Ed., Academic Press (1972) 689-710.
Vanselow, R. and Scheffler, H.-P., Convergence Analysis of a Finite Volume Method via a New Nonconforming Finite Element Method. Numer. Methods Partial Differential Equations 14 (1998) 213-231. 3.0.CO;2-R>CrossRef
R. Vanselow, Convergence Analysis for an Exponentially Fitted FVM. Preprint MATH-NM-09-99, TU Dresden (1999).
Xu, J. and Zikatanov, L., Monotone Finite El, Aement Scheme for Convection-Diffusion Equations. Math. Comp. 68 (1999) 1429-1446. CrossRef