Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Barbu, Viorel
Havârneanu, Teodor
Popa, Cătălin
and
Sritharan, S. S.
2003.
Exact controllability for the magnetohydrodynamic equations.
Communications on Pure and Applied Mathematics,
Vol. 56,
Issue. 6,
p.
732.
Barbu, Viorel
and
Lefter, Cătălin
2003.
Internal stabilizability of the Navier–Stokes equations.
Systems & Control Letters,
Vol. 48,
Issue. 3-4,
p.
161.
Barbu, Viorel
2003.
Feedback stabilization of Navier–Stokes equations.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 9,
Issue. ,
p.
197.
Wang, Gengsheng
2003.
Stabilization of the Boussinesq equation via internal feedback controls.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 52,
Issue. 2,
p.
485.
Fernández-Cara, E.
Guerrero, S.
Imanuvilov, O.Yu.
and
Puel, J.-P.
2004.
Local exact controllability of the Navier–Stokes system.
Journal de Mathématiques Pures et Appliquées,
Vol. 83,
Issue. 12,
p.
1501.
Fourestey, Gilles
and
Moubachir, Marwan
2005.
Solving inverse problems involving the Navier–Stokes equations discretized by a Lagrange–Galerkin method.
Computer Methods in Applied Mechanics and Engineering,
Vol. 194,
Issue. 6-8,
p.
877.
Fernández-Cara, Enrique
Guerrero, Sergio
Imanuvilov, Oleg Yurievich
and
Puel, Jean-Pierre
2005.
On the controllability of the N-dimensional Navier–Stokes and Boussinesq systems with N−1 scalar controls.
Comptes Rendus. Mathématique,
Vol. 340,
Issue. 4,
p.
275.
DOUBOVA, ANNA
and
FERNÁNDEZ-CARA, ENRIQUE
2005.
SOME CONTROL RESULTS FOR SIMPLIFIED ONE-DIMENSIONAL MODELS OF FLUID-SOLID INTERACTION.
Mathematical Models and Methods in Applied Sciences,
Vol. 15,
Issue. 05,
p.
783.
Fernández-Cara, Enrique
Garcia, Galina C.
and
Osses, Axel
2005.
Controls Insensitizing the Observation of a Quasi-geostrophic Ocean Model.
SIAM Journal on Control and Optimization,
Vol. 43,
Issue. 5,
p.
1616.
Bardos, Claude
2006.
Control of Fluid Flow.
Vol. 330,
Issue. ,
p.
139.
Guerrero, S.
2006.
Local exact controllability to the trajectories of the Boussinesq system.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 23,
Issue. 1,
p.
29.
Havârneanu, Teodor
Popa, Cătălin
and
Sritharan, S.S.
2006.
Exact internal controllability for the two-dimensional Navier–Stokes equations with the Navier slip boundary conditions.
Systems & Control Letters,
Vol. 55,
Issue. 12,
p.
1022.
Fernández‐Cara, Enrique
and
Guerrero, Sergio
2006.
Global Carleman Inequalities for Parabolic Systems and Applications to Controllability.
SIAM Journal on Control and Optimization,
Vol. 45,
Issue. 4,
p.
1395.
Boulakia, Muriel
and
Osses, Axel
2006.
Two-dimensional local null controllability of a rigid structure in a Navier–Stokes fluid.
Comptes Rendus. Mathématique,
Vol. 343,
Issue. 2,
p.
105.
Guerrero, Sergio
2006.
Local exact controllability to the trajectories of the Navier-Stokes system with nonlinear Navier-slip boundary conditions.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 12,
Issue. 3,
p.
484.
Fernández-Cara, Enrique
Guerrero, Sergio
Imanuvilov, Oleg Yu.
and
Puel, Jean-Pierre
2006.
Some Controllability Results forthe N-Dimensional Navier--Stokes and Boussinesq systems with N-1 scalar controls.
SIAM Journal on Control and Optimization,
Vol. 45,
Issue. 1,
p.
146.
Havârneanu, Teodor
Popa, Cătălin
and
Sritharan, S. S.
2007.
Exact Internal Controllability for the Two-Dimensional Magnetohydrodynamic Equations.
SIAM Journal on Control and Optimization,
Vol. 46,
Issue. 5,
p.
1802.
Guerrero, Sergio
2007.
Controllability of systems of Stokes equations with one control force: existence of insensitizing controls.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 24,
Issue. 6,
p.
1029.
Medjo, T. Tachim
Temam, R.
and
Ziane, M.
2008.
Optimal and Robust Control of Fluid Flows: Some Theoretical and Computational Aspects.
Applied Mechanics Reviews,
Vol. 61,
Issue. 1,
Horsin, Thierry
2008.
Local exact Lagrangian controllability of the Burgers viscous equation.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 25,
Issue. 2,
p.
219.
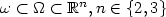 .
The result that we obtained in this paper is
as follows. Suppose that $\hat v(t,x)$
.
The result that we obtained in this paper is
as follows. Suppose that $\hat v(t,x)$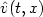 is a given
solution of the
Navier-Stokes equations. Let $ v_0(x)$
is a given
solution of the
Navier-Stokes equations. Let $ v_0(x)$ be a given initial condition
and $\Vert \hat v(0,\cdot) - v_0 \Vert < \varepsilon$
be a given initial condition
and $\Vert \hat v(0,\cdot) - v_0 \Vert < \varepsilon$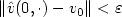 where ε is small enough. Then there
exists a locally distributed control $u,
\text{supp}\, u\subset (0,T)\times \omega
$
where ε is small enough. Then there
exists a locally distributed control $u,
\text{supp}\, u\subset (0,T)\times \omega
$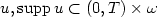 such that the solution v(t,x) of
the Navier-Stokes equations:
$$
\partial_tv-\Delta v+(v,\nabla)v=\nabla p+u+f,
\,\, \text{\rm div}\, v=0,\,\, v\vert_{\partial\Omega}=0,
\,\, v \vert_{t=0} = v_0
$$
such that the solution v(t,x) of
the Navier-Stokes equations:
$$
\partial_tv-\Delta v+(v,\nabla)v=\nabla p+u+f,
\,\, \text{\rm div}\, v=0,\,\, v\vert_{\partial\Omega}=0,
\,\, v \vert_{t=0} = v_0
$$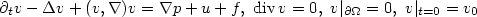 coincides with $\hat v(t,x)$
coincides with $\hat v(t,x)$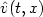 at the instant T : $v(T,x) \equiv \hat v(T,x)$
at the instant T : $v(T,x) \equiv \hat v(T,x)$ .
.