Published online by Cambridge University Press: 24 March 2010
We consider the following classical autonomous variational problem
\[ \textrm{minimize\,} \left\{F(v)=\int_a^b f(v(x),v'(x))\ \dx\,:\,v\in AC([a,b]), \;v(a)=\alpha,\; v(b)=\beta\right\},\] 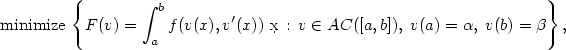
where the Lagrangian f is possibly neither continuous, nor convex, nor coercive.We prove a monotonicity property of the minimizers stating that they satisfy the maximum principle or the minimum one. By virtue of such a property, applying recent results concerning constrained variational problems, we derive a relaxation theorem, the DuBois-Reymond necessary condition and some existenceor non-existence criteria.