Hostname: page-component-76c49bb84f-hwk5l Total loading time: 0 Render date: 2025-07-13T03:30:53.901Z Has data issue: false hasContentIssue false
  • English
  • Français

Integral representation and Γ-convergence of variational integrals with p(x)-growth

Published online by Cambridge University Press:  15 September 2002

Alessandra Coscia  and
Domenico Mucci
Alessandra Coscia
Affiliation:
Dipartimento di Matematica, Università di Parma, via M. D'Azeglio 85/A, 43100 Parma, Italy; domenico.mucci@unipr.it.
Domenico Mucci
Affiliation:
Dipartimento di Matematica, Università di Parma, via M. D'Azeglio 85/A, 43100 Parma, Italy; domenico.mucci@unipr.it.
Get access

Abstract

We study the integral representation properties of limits of sequences of integral functionals like  $\int f(x,Du)\,{\rm d}x$  under nonstandard growth conditions of (p,q)-type: namely, we assume that $$ \vert z\vert^{p(x)}\leq f(x,z)\leq L(1+\vert z\vert^{p(x)})\,. $$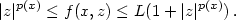 Under weak assumptions on the continuous function p(x), we prove Γ-convergence to integral functionals of the same type. We also analyse the case of integrands f(x,u,Du) depending explicitly on u; finally we weaken the assumption allowing p(x) to be discontinuous on nice sets.

Keywords

Integral representationΓ-convergencenonstandard growth conditions.

Information

Type
Research Article
Information
ESAIM: Control, Optimisation and Calculus of Variations , Volume 7 , 2002 , pp. 495 - 519
Copyright
© EDP Sciences, SMAI, 2002

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

E. Acerbi, G. Bouchitté and I. Fonseca, Relaxation of convex functionals: The gap phenomenon. Ann. Inst. H. Poincaré (2003).
Acerbi, E. and Mingione, G., Regularity results for a class of functionals with non standard growth. Arch. Rational Mech. Anal. 156 (2001) 121-140. CrossRef
E. Acerbi and G. Mingione, Regularity results for a class of quasiconvex functionals with non standard growth. Ann. Scuola Norm. Sup. Pisa Cl. Sci. 4 XXX (2001) 311-339.
R.A. Adams, Sobolev spaces. Academic Press, New York (1975).
Alkutov, Yu.A., The Harnack inequality and the Hölder property of solutions of nonlinear elliptic equations with a nonstandard growth condition. Differential Equations 33 (1998) 1653-1663.
Bouchitté, G., Fonseca, I. and Malý, J., The effective bulk energy of the relaxed energy of multiple integrals below the growth exponent. Proc. Roy. Soc. Edinburgh Ser. A 128 (1988) 463-479. CrossRef
G. Buttazzo, Semicontinuity, relaxation and integral representation in the calculus of variations. Longman, Harlow, Pitman Res. Notes in Math. 207 (1989).
Buttazzo, G. and Dal Maso, G., A characterization of nonlinear functionals on Sobolev spaces which admit an integral representation with a Carathéodory integrand. J. Math. Pures Appl. 64 (1985) 337-361.
Buttazzo, G. and Dal Maso, G., Integral representation and relaxation of local functionals. Nonlinear Anal. 9 (1985) 515-532. CrossRef
A. Braides and A. Defranceschi, Homogenization of multiple integrals. Oxford University Press, Oxford, Oxford Lecture Ser. in Maths. and its Appl. 12 (1998).
Carbone, L. and Sbordone, C., Some properties of Γ-limits of integral functionals. Ann. Mat. Pura Appl. (iv) 122 (1979) 1-60. CrossRef
Chiadò Piat, V. and Coscia, A., Hölder continuity of minimizers of functionals with variable growth exponent. Manuscripta Math. 93 (1997) 283-299. CrossRef
Coscia, A. and Mingione, G., Hölder continuity of the gradient of p(x)-harmonic mappings. C. R. Acad. Sci. Paris 328 (1999) 363-368. CrossRef
G. Dal Maso, An introduction to Γ-convergence. Birkäuser, Boston, Prog. Nonlinear Differential Equations Appl. 8 (1993).
G. Dal Maso and L. Modica, A general theory for variational functionals. Quaderno S.N.S. Pisa, Topics in Funct. Anal. (1982).
De Giorgi, E., Sulla convergenza di alcune successioni di integrali di tipo dell'area. Rend. Mat. Univ. Roma 8 (1975) 277-294.
De Giorgi, E. and Franzoni, T., Su un tipo di convergenza variazionale, Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat. 58 (1975) 842-850.
De Giorgi, E. and Letta, G., Une notion générale de convergence faible pour des fonctions croissantes d'ensemble. Ann. Scuola Norm. Sup. Pisa Cl. Sci. 4 (1977) 61-99.
I. Ekeland and R. Temam, Convex analysis and variational problems. North Holland, Amsterdam (1978).
Fan, X. and Zhao, D., A class of De Giorgi type and Hölder continuity. Nonlinear Anal. T.M.A. 36 (1999) 295-318. CrossRef
Fusco, N., On the convergence of integral functionals depending on vector-valued functions. Ricerche Mat. 32 (1983) 321-339.
Marcellini, P., Regularity and existence of solutions of elliptic equations with p,q-growth conditions. J. Differential Equations 90 (1991) 1-30. CrossRef
Marcellini, P., Regularity for some scalar variational problems under general growth conditions. J. Optim. Theory Appl. 90 (1996) 161-181. CrossRef
Morrey, C.B., Quasi-convexity and semicontinuity of multiple integrals. Pacific J. Math. 2 (1952) 25-53. CrossRef
Rajagopal, K.R. and M. $\rm R{\mathaccent'27u}{\mathaccent'24z}i{\mathaccent'24c}ka$ , Mathematical modelling of electrorheological fluids. Cont. Mech. Therm. 13 (2001) 59-78. CrossRef
M. $\rm R{\mathaccent'27u}{\mathaccent'24z}i{\mathaccent'24c}ka$ , Electrorheological fluids: Modeling and mathematical theory. Springer, Berlin, Lecture Notes in Math. 1748 (2000).
Zhikov, V.V., On the passage to the limit in nonlinear variational problems. Russian Acad. Sci. Sb. Math. 76 (1993) 427-459.
Zhikov, V.V., Lavrentiev's, On phenomenon. Russian J. Math. Phys. 3 (1995) 249-269.
Zhikov, V.V., On some variational problems. Russian J. Math. Phys. 5 (1997) 105-116.
Zhikov, V.V., Meyers type estimates for solving the non linear Stokes system. Differential Equations 33 (1997) 107-114.
V.V. Zhikov, S.M. Kozlov and O.A. Oleinik, Homogenization of differential operators and integral functionals. Springer, Berlin (1994).