Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Fernández‐Cara, Enrique
and
Guerrero, Sergio
2006.
Global Carleman Inequalities for Parabolic Systems and Applications to Controllability.
SIAM Journal on Control and Optimization,
Vol. 45,
Issue. 4,
p.
1395.
Clason, Christian
and
Hepperger, Peter
2009.
A Forward Approach to Numerical Data Assimilation.
SIAM Journal on Scientific Computing,
Vol. 31,
Issue. 4,
p.
3090.
Thevenet, Laetitia
Buchot, Jean-Marie
and
Raymond, Jean-Pierre
2010.
Nonlinear feedback stabilization of a two-dimensional Burgers equation.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 16,
Issue. 4,
p.
929.
Maniar, Lahcen
Meyries, Martin
and
Schnaubelt, Roland
2017.
Null controllability for parabolic equations with dynamic boundary conditions.
Evolution Equations & Control Theory,
Vol. 6,
Issue. 3,
p.
381.
Qingzhe, Wu
and
Peidong, Lei
2018.
Multiplicative controllability of heat equations with the homogeneous Neumann boundary condition.
Mathematical Methods in the Applied Sciences,
Vol. 41,
Issue. 2,
p.
472.
Ziane, Tarik Ali
Ouzzane, Hadjer
and
Zair, Ouahiba
2018.
Controllability results for the two-dimensional heat equation with mixed boundary conditions using Carleman inequalities: a linear and a semilinear case.
Applicable Analysis,
Vol. 97,
Issue. 14,
p.
2412.
Okposo, Newton
and
Willie, Robert
2019.
Well-posedness, blow-up dynamics and controllability of the classical chemotaxis model.
Advances in Pure and Applied Mathematics,
Vol. 10,
Issue. 2,
p.
93.
Le Balc'h, Kévin
2020.
Global null-controllability and nonnegative-controllability of slightly superlinear heat equations.
Journal de Mathématiques Pures et Appliquées,
Vol. 135,
Issue. ,
p.
103.
Kassab, K.
2020.
Null controllability of semi-linear fourth order parabolic equations.
Journal de Mathématiques Pures et Appliquées,
Vol. 136,
Issue. ,
p.
279.
Le Rousseau, Jérôme
Lebeau, Gilles
and
Robbiano, Luc
2022.
Elliptic Carleman Estimates and Applications to Stabilization and Controllability, Volume I.
Vol. 97,
Issue. ,
p.
251.
Wang, Chunpeng
and
Zhou, Yanan
2023.
Carleman estimate and null controllability for a degenerate parabolic equation with a slightly superlinear reaction term.
Nonlinear Differential Equations and Applications NoDEA,
Vol. 30,
Issue. 5,
Boulite, Said
Elgrou, Abdellatif
and
Maniar, Lahcen
2025.
Null controllability for stochastic parabolic equations with Robin boundary conditions.
Evolution Equations and Control Theory,
Vol. 14,
Issue. 5,
p.
990.
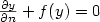 . We consider distributed controls, with support in a small set. The null controllability of similar linear systems has been analyzedin a previous first part of this work. In this second part we show that, when the nonlinear terms arelocally Lipschitz-continuous and slightly superlinear, one has exactcontrollability to the trajectories.
. We consider distributed controls, with support in a small set. The null controllability of similar linear systems has been analyzedin a previous first part of this work. In this second part we show that, when the nonlinear terms arelocally Lipschitz-continuous and slightly superlinear, one has exactcontrollability to the trajectories.