Article contents
Weak ergodic averages over dilated measures
Published online by Cambridge University Press: 07 October 2019
Abstract
Let 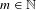 $m\in \mathbb{N}$ and
$m\in \mathbb{N}$ and 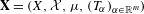 $\mathbf{X}=(X,{\mathcal{X}},\unicode[STIX]{x1D707},(T_{\unicode[STIX]{x1D6FC}})_{\unicode[STIX]{x1D6FC}\in \mathbb{R}^{m}})$ be a measure-preserving system with an
$\mathbf{X}=(X,{\mathcal{X}},\unicode[STIX]{x1D707},(T_{\unicode[STIX]{x1D6FC}})_{\unicode[STIX]{x1D6FC}\in \mathbb{R}^{m}})$ be a measure-preserving system with an 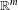 $\mathbb{R}^{m}$ -action. We say that a Borel measure
$\mathbb{R}^{m}$ -action. We say that a Borel measure  $\unicode[STIX]{x1D708}$ on
$\unicode[STIX]{x1D708}$ on 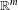 $\mathbb{R}^{m}$ is weakly equidistributed for
$\mathbb{R}^{m}$ is weakly equidistributed for 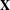 $\mathbf{X}$ if there exists
$\mathbf{X}$ if there exists 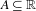 $A\subseteq \mathbb{R}$ of density 1 such that, for all
$A\subseteq \mathbb{R}$ of density 1 such that, for all 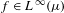 $f\in L^{\infty }(\unicode[STIX]{x1D707})$ , we have
$f\in L^{\infty }(\unicode[STIX]{x1D707})$ , we have  $$\begin{eqnarray}\lim _{t\in A,t\rightarrow \infty }\int _{\mathbb{R}^{m}}f(T_{t\unicode[STIX]{x1D6FC}}x)\,d\unicode[STIX]{x1D708}(\unicode[STIX]{x1D6FC})=\int _{X}f\,d\unicode[STIX]{x1D707}\end{eqnarray}$$
$$\begin{eqnarray}\lim _{t\in A,t\rightarrow \infty }\int _{\mathbb{R}^{m}}f(T_{t\unicode[STIX]{x1D6FC}}x)\,d\unicode[STIX]{x1D708}(\unicode[STIX]{x1D6FC})=\int _{X}f\,d\unicode[STIX]{x1D707}\end{eqnarray}$$ $\unicode[STIX]{x1D707}$ -almost every
$\unicode[STIX]{x1D707}$ -almost every  $x\in X$ . Let
$x\in X$ . Let 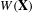 $W(\mathbf{X})$ denote the collection of all
$W(\mathbf{X})$ denote the collection of all  $\unicode[STIX]{x1D6FC}\in \mathbb{R}^{m}$ such that the
$\unicode[STIX]{x1D6FC}\in \mathbb{R}^{m}$ such that the  $\mathbb{R}$ -action
$\mathbb{R}$ -action 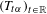 $(T_{t\unicode[STIX]{x1D6FC}})_{t\in \mathbb{R}}$ is not ergodic. Under the assumption of the pointwise convergence of the double Birkhoff ergodic average, we show that a Borel measure
$(T_{t\unicode[STIX]{x1D6FC}})_{t\in \mathbb{R}}$ is not ergodic. Under the assumption of the pointwise convergence of the double Birkhoff ergodic average, we show that a Borel measure  $\unicode[STIX]{x1D708}$ on
$\unicode[STIX]{x1D708}$ on 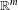 $\mathbb{R}^{m}$ is weakly equidistributed for an ergodic system
$\mathbb{R}^{m}$ is weakly equidistributed for an ergodic system  $\mathbf{X}$ if and only if
$\mathbf{X}$ if and only if  $\unicode[STIX]{x1D708}(W(\mathbf{X})+\unicode[STIX]{x1D6FD})=0$ for every
$\unicode[STIX]{x1D708}(W(\mathbf{X})+\unicode[STIX]{x1D6FD})=0$ for every 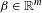 $\unicode[STIX]{x1D6FD}\in \mathbb{R}^{m}$ . Under the same assumption, we also show that
$\unicode[STIX]{x1D6FD}\in \mathbb{R}^{m}$ . Under the same assumption, we also show that  $\unicode[STIX]{x1D708}$ is weakly equidistributed for all ergodic measure-preserving systems with
$\unicode[STIX]{x1D708}$ is weakly equidistributed for all ergodic measure-preserving systems with 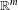 $\mathbb{R}^{m}$ -actions if and only if
$\mathbb{R}^{m}$ -actions if and only if 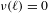 $\unicode[STIX]{x1D708}(\ell )=0$ for all hyperplanes
$\unicode[STIX]{x1D708}(\ell )=0$ for all hyperplanes 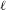 $\ell$ of
$\ell$ of  $\mathbb{R}^{m}$ . Unlike many equidistribution results in literature whose proofs use methods from harmonic analysis, our results adopt a purely ergodic-theoretic approach.
$\mathbb{R}^{m}$ . Unlike many equidistribution results in literature whose proofs use methods from harmonic analysis, our results adopt a purely ergodic-theoretic approach.
MSC classification
Information
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2019
References
- 3
- Cited by


