Article contents
A strongly irreducible affine iterated function system with two invariant measures of maximal dimension
Published online by Cambridge University Press: 30 October 2020
Abstract
A classical theorem of Hutchinson asserts that if an iterated function system acts on  $\mathbb {R}^{d}$ by similitudes and satisfies the open set condition then it admits a unique self-similar measure with Hausdorff dimension equal to the dimension of the attractor. In the class of measures on the attractor, which arise as the projections of shift-invariant measures on the coding space, this self-similar measure is the unique measure of maximal dimension. In the context of affine iterated function systems it is known that there may be multiple shift-invariant measures of maximal dimension if the linear parts of the affinities share a common invariant subspace, or more generally if they preserve a finite union of proper subspaces of
$\mathbb {R}^{d}$ by similitudes and satisfies the open set condition then it admits a unique self-similar measure with Hausdorff dimension equal to the dimension of the attractor. In the class of measures on the attractor, which arise as the projections of shift-invariant measures on the coding space, this self-similar measure is the unique measure of maximal dimension. In the context of affine iterated function systems it is known that there may be multiple shift-invariant measures of maximal dimension if the linear parts of the affinities share a common invariant subspace, or more generally if they preserve a finite union of proper subspaces of 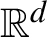 $\mathbb {R}^{d}$. In this paper we give an example where multiple invariant measures of maximal dimension exist even though the linear parts of the affinities do not preserve a finite union of proper subspaces.
$\mathbb {R}^{d}$. In this paper we give an example where multiple invariant measures of maximal dimension exist even though the linear parts of the affinities do not preserve a finite union of proper subspaces.
Keywords
MSC classification
Information
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 3
- Cited by


