Article contents
Smale endomorphisms over graph-directed Markov systems
Published online by Cambridge University Press: 08 June 2020
Abstract
We study Smale skew product endomorphisms (introduced in Mihailescu and Urbański [Skew product Smale endomorphisms over countable shifts of finite type. Ergod. Th. & Dynam. Sys. doi: 10.1017/etds.2019.31. Published online June 2019]) now over countable graph-directed Markov systems, and we prove the exact dimensionality of conditional measures in fibers, and then the global exact dimensionality of the equilibrium measure itself. Our results apply to large classes of systems and have many applications. They apply, for instance, to natural extensions of graph-directed Markov systems. Another application is to skew products over parabolic systems. We also give applications in ergodic number theory, for example to the continued fraction expansion, and the backward fraction expansion. In the end we obtain a general formula for the Hausdorff (and pointwise) dimension of equilibrium measures with respect to the induced maps of natural extensions 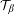 ${\mathcal{T}}_{\unicode[STIX]{x1D6FD}}$ of
${\mathcal{T}}_{\unicode[STIX]{x1D6FD}}$ of  $\unicode[STIX]{x1D6FD}$-maps
$\unicode[STIX]{x1D6FD}$-maps 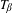 $T_{\unicode[STIX]{x1D6FD}}$, for arbitrary
$T_{\unicode[STIX]{x1D6FD}}$, for arbitrary  $\unicode[STIX]{x1D6FD}>1$.
$\unicode[STIX]{x1D6FD}>1$.
Keywords
MSC classification
Information
- Type
- Original Article
- Information
- Copyright
- © The Author(s) 2020. Published by Cambridge University Press
References
- 1
- Cited by


