Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Eisner, Tanja
Farkas, Bálint
Haase, Markus
and
Nagel, Rainer
2015.
Operator Theoretic Aspects of Ergodic Theory.
Vol. 272,
Issue. ,
p.
161.
Kunde, Philipp
2015.
Uniform rigidity sequences for weak mixing diffeomorphisms onD2,AandT2.
Journal of Mathematical Analysis and Applications,
Vol. 429,
Issue. 1,
p.
111.
Adams, Terrence
and
Silva, Cesar E.
2018.
Ergodic Theory and Dynamical Systems in their Interactions with Arithmetics and Combinatorics.
Vol. 2213,
Issue. ,
p.
327.
Kunde, Philipp
2018.
Uniform rigidity sequences for weakly mixing diffeomorphisms on Dm, Tm and S1×[0,1]m−1.
Journal of Mathematical Analysis and Applications,
Vol. 462,
Issue. 2,
p.
1398.
GRIESMER, JOHN T.
2019.
Recurrence, rigidity, and popular differences.
Ergodic Theory and Dynamical Systems,
Vol. 39,
Issue. 5,
p.
1299.
Cuny, Christophe
Eisner, Tanja
and
Farkas, Bálint
2019.
Wiener's lemma along primes and other subsequences.
Advances in Mathematics,
Vol. 347,
Issue. ,
p.
340.
ROBERTSON, DONALD
2019.
Mild mixing of certain interval-exchange transformations.
Ergodic Theory and Dynamical Systems,
Vol. 39,
Issue. 1,
p.
248.
Frączek, Krzysztof
2019.
Ergodic properties of the ideal gas model for infinite billiards.
Physica D: Nonlinear Phenomena,
Vol. 390,
Issue. ,
p.
9.
Cai, Fangzhou
2020.
Measure-theoretic equicontinuity and rigidity.
Nonlinearity,
Vol. 33,
Issue. 8,
p.
3739.
LE, ANH NGOC
2020.
Nilsequences and multiple correlations along subsequences.
Ergodic Theory and Dynamical Systems,
Vol. 40,
Issue. 6,
p.
1634.
Kanigowski, Adam
and
Lemańczyk, Mariusz
2020.
Encyclopedia of Complexity and Systems Science.
p.
1.
Badea, Catalin
Grivaux, Sophie
and
Matheron, Étienne
2021.
Rigidity sequences, Kazhdan sets and group topologies on the integers.
Journal d'Analyse Mathématique,
Vol. 143,
Issue. 1,
p.
313.
Podvigin, I. V.
2022.
On Possible Estimates of the Rate of Pointwise Convergence in the Birkhoff Ergodic Theorem.
Siberian Mathematical Journal,
Vol. 63,
Issue. 2,
p.
316.
Ackelsberg, Ethan M.
2022.
Rigidity, weak mixing, and recurrence in abelian groups.
Discrete & Continuous Dynamical Systems,
Vol. 42,
Issue. 4,
p.
1669.
Adams, Terrence
and
Quas, Anthony
2022.
Encyclopedia of Complexity and Systems Science.
p.
1.
Haddock, Beatrix
Leng, James
and
Silva, Cesar E.
2022.
Nonsingular transformations that are ergodic with isometric coefficients and not weakly doubly ergodic.
Indagationes Mathematicae,
Vol. 33,
Issue. 6,
p.
1297.
JAMNESHAN, ASGAR
and
TAO, TERENCE
2023.
An uncountable Moore–Schmidt theorem.
Ergodic Theory and Dynamical Systems,
Vol. 43,
Issue. 7,
p.
2376.
Kanigowski, Adam
and
Lemańczyk, Mariusz
2023.
Ergodic Theory.
p.
109.
Adams, Terrence
and
Quas, Anthony
2023.
Ergodic Theory.
p.
35.
ZELADA, RIGOBERTO
2023.
Mixing and rigidity along asymptotically linearly independent sequences.
Ergodic Theory and Dynamical Systems,
Vol. 43,
Issue. 10,
p.
3506.
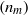 $({n}_{m} )$ of positive integers, namely rigidity and non-recurrence. Our goal is to find conditions on the sequence which ensure that it is, or is not, a rigid sequence or a non-recurrent sequence for some weakly mixing system or more generally for some ergodic system. The main focus is on weakly mixing systems. For example, we show that for any integer
$({n}_{m} )$ of positive integers, namely rigidity and non-recurrence. Our goal is to find conditions on the sequence which ensure that it is, or is not, a rigid sequence or a non-recurrent sequence for some weakly mixing system or more generally for some ergodic system. The main focus is on weakly mixing systems. For example, we show that for any integer 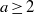 $a\geq 2$ the sequence
$a\geq 2$ the sequence 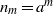 ${n}_{m} = {a}^{m} $ is a sequence of rigidity for some weakly mixing system. We show the same for the sequence of denominators of the convergents in the continued fraction expansion of any irrational
${n}_{m} = {a}^{m} $ is a sequence of rigidity for some weakly mixing system. We show the same for the sequence of denominators of the convergents in the continued fraction expansion of any irrational  $\alpha $. We also consider the stronger property of IP-rigidity. We show that if
$\alpha $. We also consider the stronger property of IP-rigidity. We show that if 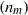 $({n}_{m} )$ grows fast enough then there is a weakly mixing system which is IP-rigid along
$({n}_{m} )$ grows fast enough then there is a weakly mixing system which is IP-rigid along  $({n}_{m} )$ and non-recurrent along
$({n}_{m} )$ and non-recurrent along 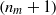 $({n}_{m} + 1)$.
$({n}_{m} + 1)$.
